NCERT Solutions for Class 10 Maths Chapter 11 Constructions

NCERT Solutions Class 10 Maths Chapter 11 Constructions – Here are all the NCERT solutions for Class 10 Maths Chapter 11. This solution contains questions, answers, images, explanations of the complete Chapter 11 titled Constructions of Maths taught in Class 10. If you are a student of Class 10 who is using NCERT Textbook to study Maths, then you must come across Chapter 11 Constructions. After you have studied lesson, you must be looking for answers of its questions. Here you can get complete NCERT Solutions for Class 10 Maths Chapter 11 Constructions in one place.

Go to Exercise

NCERT Solutions Class 10 Maths Chapter 11 Constructions

Here on AglaSem Schools, you can access to NCERT Book Solutions in free pdf for Maths for Class 10 so that you can refer them as and when required. The NCERT Solutions to the questions after every unit of NCERT textbooks aimed at helping students solving difficult questions.

For a better understanding of this chapter, you should also see summary of Chapter 11 Constructions , Maths, Class 10.

Class	10
Subject	Maths
Book	Mathematics
Chapter Number	11
Chapter Name	Constructions

NCERT Solutions Class 10 Maths chapter 11 Constructions

Class 10, Maths chapter 11, Constructions solutions are given below in PDF format. You can view them online or download PDF file for future use.

Constructions Download

NCERT Solutions for Class 10 Maths Chapter 11 Constructions

Did you find NCERT Solutions Class 10 Maths chapter 11 Constructions helpful? If yes, please comment below. Also please like, and share it with your friends!

NCERT Solutions Class 10 Maths chapter 11 Constructions- Video

You can also watch the video solutions of NCERT Class10 Maths chapter 11 Constructions here.

If you liked the video, please subscribe to our YouTube channel so that you can get more such interesting and useful study resources.

Download NCERT Solutions Class 10 Maths chapter 11 Constructions In PDF Format

You can also download here the NCERT Solutions Class 10 Maths chapter 11 Constructions in PDF format.

Click Here to download NCERT Solutions for Class 10 Maths chapter 11 Constructions

Question & Answer

Q.1: In the question, give the justification of the construction also Draw a line segment of length 7.6 cm and divide it in the ratio 5 : 8. Measure the two parts.

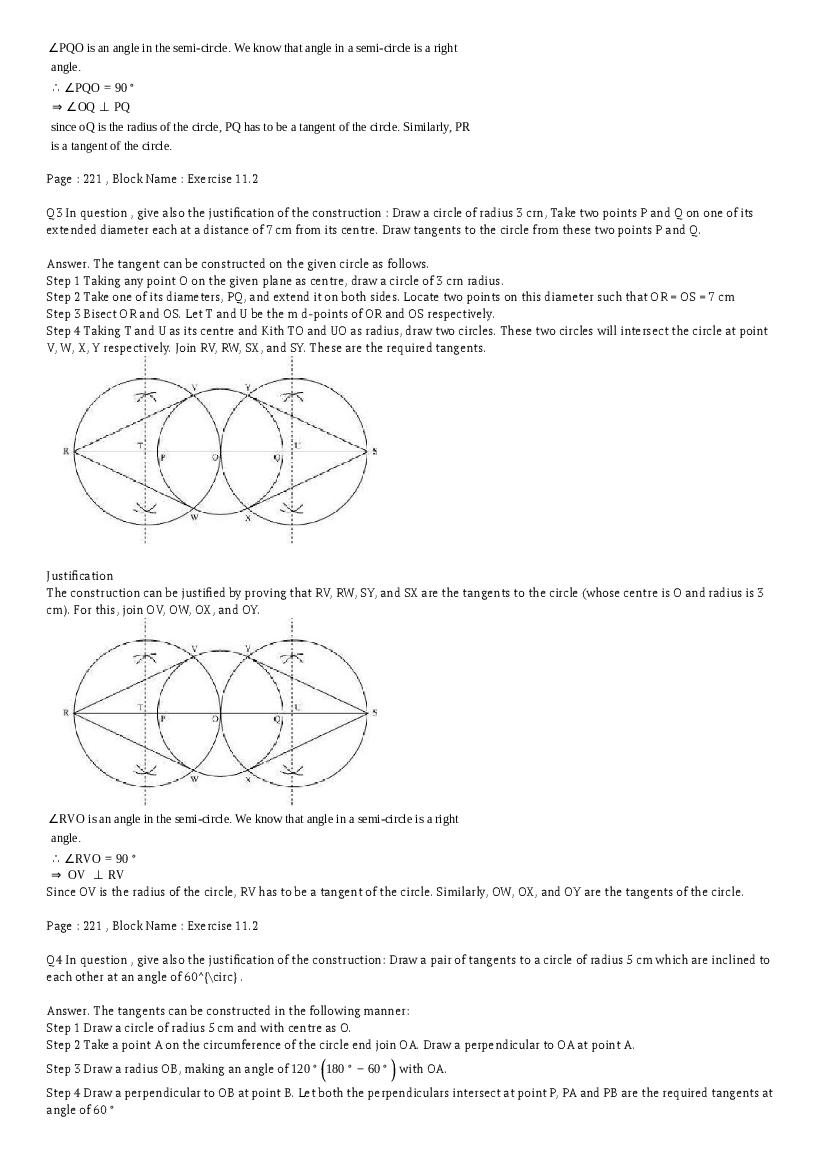
Ans : A line segment of length 7.6 cm can be divided in the ratio of 5 : 8 as follows
Step 1 Draw line segment AB of 7.6 cm and draw a ray AX making an acute angle with line segment AB .
Step 2 Locate L3=(= 5+8) points, \(A_{1}, A_{2}, A_{3}, A_{4}, …..A_{13},\) on AX such that \(AA_{1}=A_{1}A_{2}=A_{2}A_{3}\) and so on . 
Step 3 Join \(BA_{13}\).
Step 4 Through the point \(A_{5}\), draw a line parallel to \(BA_{13}\) (by making an angle equal to \(\angle A A_{13} B\)) at \(A_{5}\) intersecting AB at point C.
C is the point dividing line segment AB of 7.6 cm in the required ratio of 5 : 8.
The lengths of AC and CB can be measured. It comes out to 2.9 cm and 4.7 cm respectively.



Justification
The construction can be justified by proving that 
\(\begin{array}{l}{\frac{\mathrm{AC}}{\mathrm{CB}}=\frac{5}{8}} \\ {\text { By construction, we have } \mathrm{A}_{5} \mathrm{C}\left\|\mathrm{A}_{13} \mathrm{B} \text { . By applying Basic proportionality theorem for }\right.} \\ {\frac{\mathrm{AC}}{\mathrm{CB}}=\frac{\mathrm{AA}_{5}}{\mathrm{A}_{5} \mathrm{A}_{13}}}\end{array}\)
\(\begin{array}{l}{\text { From the figure, it can be observed that } A A_{5} \text { and } A_{5} A_{13} \text { contain } 5 \text { and } 8 \text { equal }} \\ {\text { divisions of line segments respectively. }} \\ {\therefore \frac{A A_{f}}{A_{3} A_{13}}=\frac{5}{8}} \\ {\text { On comparing equations }(1) \text { and }(2), \text { we obtain }} \\ {\frac{A C}{C B}=\frac{5}{8}} \\ {\text { This justifies the construction. }}\end{array}\) 

Q.2: In the question, give the justification of the construction also , construct a triangle of sides 4 cm, 5 cm and 6 cm and then a triangle similar to it whose sides are \(\frac{2}{3}\) of the corresponding sides of the first triangle.

Ans : Step 1 Draw a line segment AB = 4 cm. Taking point A as centre, draw and arc of 5 cm radius. Similarly, taking point B as its centre, draw an arc of 6 cm radius. These arcs will intersect each other at point C. Now, AC = 5 cm and BC=6 cm 
And \(\triangle ABC\) is the required triangle.
Step 2 Draw a ray AX  making an acute angle with line AB on the opposite site of vertex C.
Step 3 Locate 3 points (as 3 is greater between 2 and 3) online AX such that \(AA_{1}=A_{1}A_{2}=A_{2}A_{3}\).
Step 4 \(Join \mathrm{BA}_{3}\) and draw a line through \(\mathrm{A}_{2}\) parallel to \(\mathrm{B} \mathrm{A}_{3}\) to intersect \(\mathrm{AB}\) at point \(\mathrm{B}^{\prime} .\)
 Step 5  Draw a line through B\(\prime\) parallel to the line BC to intersect AC at \(C^{\prime}\) \(\triangle A B^{\prime} C^{\prime}\)is the required triangle.

Justification
The construction can be justified by proving that 
\(\mathrm{AB}^{\prime}=\frac{2}{3} \mathrm{AB}, \mathrm{B} \mathrm{C}^{\prime}=\frac{2}{3} \mathrm{BC}, \mathrm{AC}^{\prime}=\frac{2}{3} \mathrm{AC}\)
\(\begin{array}{l}{\text { By construction, we have } B^{\prime} C^{\prime}\|B C} \\ {\therefore \angle A B C^{\prime}=\angle A B C(\text {Corresponding angles) }}\end{array}\)
\(\triangle A B^{\prime} C^{\prime} \text { and } \triangle A B C\)
\(\begin{aligned} \angle \mathrm{ABC}^{\prime} &=\angle A B C(\text { Proved above }) \\ \angle \mathrm{B}^{\prime} \mathrm{AC}^{\prime} &=\angle B A C(\text { Common }) \end{aligned}\)
\(\therefore \triangle \mathrm{ABC}^{\prime}-\Delta \mathrm{ABC}(\text { AA similarity criterion })\)
\(\Rightarrow \frac{A B^{\prime}}{A B}=\frac{B^{\prime} C^{\prime}}{B C}=\frac{A C^{\prime}}{A C}\)
\(\triangle \mathrm{AA}_{2} \mathrm{B}^{\prime} \text { and } \triangle \mathrm{A} \mathrm{A}_{3} \mathrm{B}\)
\(\begin{array}{l}{\angle A_{2} A B^{\prime}=\angle A_{3} A B(\text {Corresponding} \text { angles })} \\ {\angle A A_{2} B^{\prime}=\angle A A_{3} B(\text {Corresponding angles) }}\end{array}\)
\(\begin{array}{l}{\therefore \triangle A A_{2} B^{\prime}-\Delta A A_{3} B(\text { AA similarity criterion) }} \\ {\Rightarrow \frac{A B^{\prime}}{A B}=\frac{A A_{2}}{A A_{3}}}\end{array}\)
\(\Rightarrow \frac{\mathrm{AB}^{\prime}}{\mathrm{AB}}=\frac{2}{3}\)
\(\begin{array}{l}{\text { From equations }(1) \text { and }(2), \text { we obtain }} \\ {\frac{\mathrm{AB}^{\prime}}{\mathrm{AB}}=\frac{\mathrm{B} \mathrm{C}^{\prime}}{\mathrm{BC}}=\frac{\mathrm{AC}^{\prime}}{\mathrm{AC}}=\frac{2}{3}} \\ {\Rightarrow \mathrm{AB}^{\prime}=\frac{2}{3} \mathrm{AB}, \mathrm{B}^{\prime} \mathrm{C}^{\prime}=\frac{2}{3} \mathrm{BC}, \mathrm{AC}^{\prime}=\frac{2}{3} \mathrm{AC}} \\ {\text { This justifies the construction. }}\end{array}\) 

Q.3: In the question, give the justification of the construction also ,construct a triangle with sides 5 cm, 6 cm and 7 cm and then another triangle whose sides are \(\frac{7}{5}\) of the corresponding sides of the first triangle.

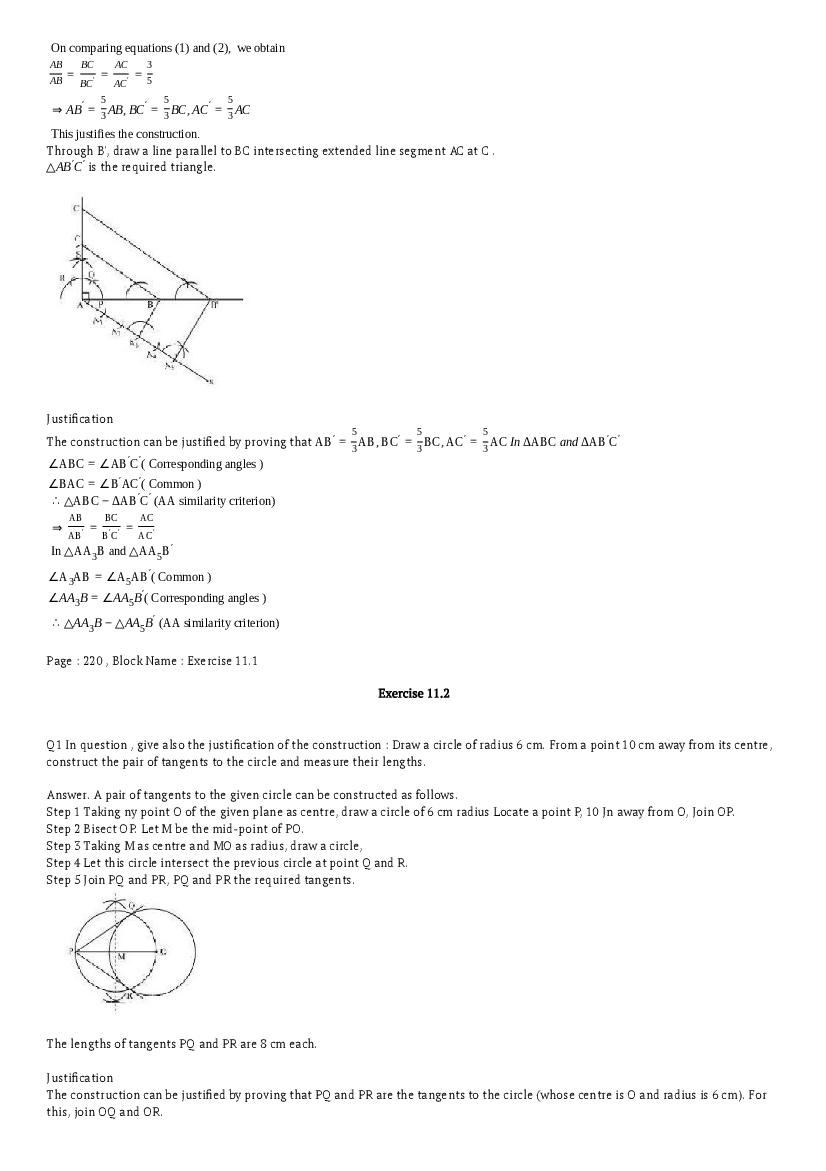
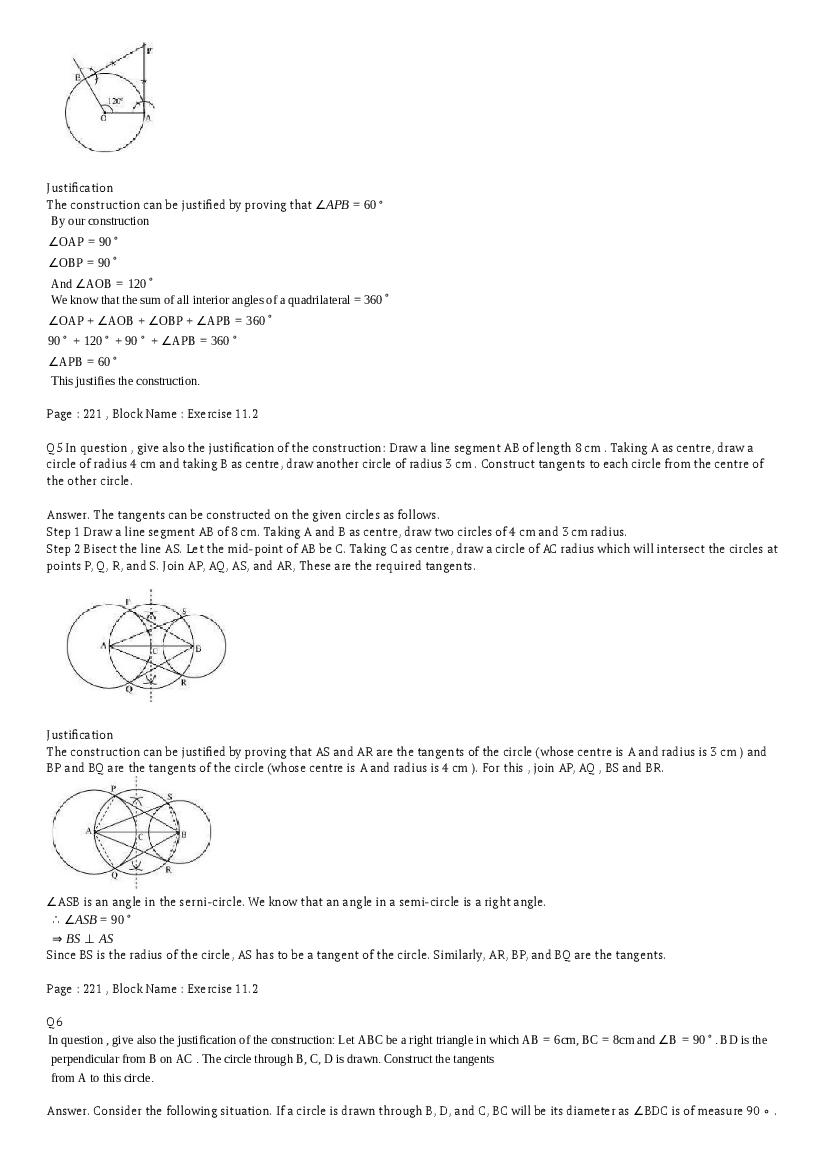
Ans : Step 1 Draw a line segment AB of 5 cm. Taking A and B as centre, draw arcs of 6 cm and 5 cm radius respectively. Let these arcs intersect each other at point C.\(\triangle ABC\) is the required triangle having length of sides as 5 cm , 6 cm , 7 cm respectively.
Step 2 Draw a ray AX making acute angle with line AB on the opposite side of vertex C.
Step 3 
\(\begin{array}{l}{\text { Locate } 7 \text { points, } A_{1}, A_{2}, A_{3}, A_{4} A_{5}, A_{5}, A_{7}(\text { as } 7 \text { is greater between } 5 \text { and } 7), \text { on line } A X} \\ {\text { such that } A A_{1}=A_{1} A_{2}=A_{2} A_{3}=A_{3} A_{4}=A_{4} A_{5}=A_{5} A_{6}=A_{6} A_{7}}\end{array}\)
Step 4 Join \(BA_{5}\) and draw a line through \(A_{7}\) parallel to \(BA_{5}\) to intersect extended line segment AB at point \(B^{\prime}\).
Step 5 Draw a line through \(B^{\prime}\) parallel to BC intersecting the extended line segment AC at \(C^{\prime}\).\(\triangle\)A\(B^{\prime}\)\(C^{\prime}\) is the required triangle.



Justification
The construction can be justified by proving that 
\(\begin{array}{l}{\mathrm{AB}^{\prime}=\frac{7}{5} \mathrm{AB}, \mathrm{B} \mathrm{C}^{\prime}=\frac{7}{5} \mathrm{BC}, \mathrm{AC}^{\prime}=\frac{7}{5} \mathrm{AC}} \\ {\text { In } \triangle \mathrm{ABC} \text { and } \Delta \mathrm{AB}^{\prime} \mathrm{C}^{\prime}} \\ {\angle \mathrm{ABC}=\angle \mathrm{AB}^{\prime} \mathrm{C}^{\prime}(\text { Corresponding angles })} \\ {\angle \mathrm{BAC}=\angle \mathrm{B}^{\prime} \mathrm{AC}^{\prime}(\text { Common })}\end{array}\)
\(\therefore \triangle \mathrm{ABC}-\triangle \mathrm{AB}^{\prime} \mathrm{C}^{\prime}\)
\(\Rightarrow \frac{\mathrm{AB}}{\mathrm{AB}^{\prime}}=\frac{\mathrm{BC}}{\mathrm{B}^{\prime} \mathrm{C}^{\prime}}=\frac{\mathrm{AC}}{\mathrm{AC}^{\prime}} \ldots\)
\(\begin{array}{l}{\text { In } \triangle A A_{5} B \text { and } \triangle A A_{7} B^{\prime}} \\ {\angle A_{5} A B=\angle A_{7} A B^{\prime}(\text { Common })}\end{array}\)
\(\angle A A_{\mathrm{s}} B=\angle A A_{7} B^{\prime}(\text { Corresponding angles })\)
\(\begin{array}{l}{\therefore \triangle \mathrm{AA}_{5} \mathrm{B}-\triangle \mathrm{AA}_{7} \mathrm{B}^{\prime} \text { (AA similarity criterion) }} \\ {\Rightarrow \frac{\mathrm{AB}}{\mathrm{AB}^{\prime}}=\frac{\mathrm{AA}_{5}}{\mathrm{AA}_{7}}} \\ {\Rightarrow \frac{\mathrm{AB}}{\mathrm{AB}^{\prime}}=\frac{5}{7}}\end{array}\)
\(\begin{array}{l}{\text { On comparing equations }(1) \text { and }(2), \text { we obtain }} \\ {\frac{\mathrm{AB}}{\mathrm{AB}^{\prime}}=\frac{\mathrm{BC}}{\mathrm{BC}^{\prime}}=\frac{\mathrm{AC}}{\mathrm{AC}^{\prime}}=\frac{5}{7}} \\ {\Rightarrow \mathrm{AB}^{\prime}=\frac{7}{5} \mathrm{AB}, \mathrm{B}^{\prime} \mathrm{C}=\frac{7}{5} \mathrm{BC}, \mathrm{AC}=\frac{7}{5} \mathrm{AC}} \\ {\text { This justifies the construction. }}\end{array}\) 

Q.4: \(\begin{array}{l}{\text { In the question, give the justification of the construction also , construct an isosceles triangle whose base is } 8 \mathrm{cm} \text { and altitude } 4 \mathrm{cm} \text { and then another }} \\ {\text { triangle whose sides are } 1 \frac{1}{2} \text { times the corresponding sides of the isosceles triangle. }}\end{array}\)

Ans : Let us assume that ∠ABC is an isosceles triangle having CA and CB of equal lengths, base AB of 8 crn, and AD is the altitude of 4 cm. \(A \triangle A B^{\prime} C^{\prime}\)whose sides are \(\frac{3}{2}\) times of can be drawn as follows.
Step 1 Draw a line segment Aa of 8 cm. Draw arcs of same radius on both sides of the line segment while taking point A and as its centre. Let these arcs intersect each other at O and O'. Join OO' Let OO' intersect AB at D.
Step 2 Taking D as centre, draw an arc of 4 cm radius which cuts the extended line segment OO' at point C. An isosceles \(AA_{3}C\) is formed, having CD (altitude) as 4 cm and AB (base) as 8 cm.
Step 3 Draw a ray AX making an acute angle with line segment  AB on the opposite side of vertex C.
Step 4 Locate 3 points (as 3 is greater between 3 and 2) \(A_{1}, A_{2}, \text { and } A_{3}\) on AX such that \(AA_{1}= A_{1}A_{2}= A_{2}A_{3}\).
Step 5 Join \(BA_{2}\) and draw a line through \(A_{3}\) parallel to \(BA_{2}\) to intersect extended line segment AB at point \(B^{\prime}\).
Step 6 Draw a line through \(B^{\prime}\) parallel to BC intersecting the extended the segment AC at \(C^{\prime}\). \(\Delta \mathrm{AB}^{\prime} \mathrm{C}^{\prime}\) Is the required triangle.



Justification
The construction can be justified by proving that
\(\mathrm{AB}^{\prime}=\frac{3}{2} \mathrm{AB}, \mathrm{BC}^{\prime}=\frac{3}{2} \mathrm{BC}, \mathrm{AC}^{\prime}=\frac{3}{2} \mathrm{AC}\)
\(\begin{array}{l}{\text { In } \triangle A B C \text { and } \Delta A B^{\prime} C^{\prime}} \\ {\angle A B C=\angle A B^{\prime} C^{\prime} \text { (Corresponding angles) }}\end{array}\)
\(\begin{array}{l}{\angle B A C=\angle B^{\prime} A C^{\prime}(\text { Common })} \\ {\therefore \triangle A B C-\Delta A B^{\prime} C^{\prime} \text { (AA similarity criterion) }}\end{array}\)
\(\begin{array}{l}{\Rightarrow \frac{A B}{A B^{\prime}}=\frac{B C}{B C^{\prime}}=\frac{A C}{A C^{\prime}}} \\ {\text { In } \triangle A A_{2} B \text { and } \Delta A A_{3} B^{\prime}}\end{array}\)
\(\begin{array}{l}{\angle A_{2} A B=\angle A_{3} A B^{\prime}(\text { Corresponding angles })} \\ {\angle A A_{2} B=\angle A A_{3} B^{\prime} \text { (Corresponding angles) }}\end{array}\)
\(\begin{array}{l}{\therefore \triangle \mathrm{AA}_{2} \mathrm{B}-\triangle \mathrm{AA}_{3} \mathrm{B}^{\prime} \text { (AA similarity criterion) }} \\ {\Rightarrow \frac{\mathrm{AB}}{\mathrm{AB}}=\frac{\mathrm{AA}_{2}}{\mathrm{AA}_{3}}} \\ {\Rightarrow \frac{\mathrm{AB}}{\mathrm{AB}^{\prime}}=\frac{2}{3}}\end{array}\)
\(\begin{array}{l}{\text { On comparing equations }(1) \text { and }(2), \text { we obtain }} \\ {\frac{A B}{A B}=\frac{B C}{B C^{\prime}}=\frac{A C}{A C^{\prime}}=\frac{2}{3}} \\ {\Rightarrow A B^{\prime}=\frac{3}{2} A B, B C=\frac{3}{2} B C, A C=\frac{3}{2} A C} \\ {\text { This justifies the construction. }}\end{array}\) 

Q.5: In the question, give the justification of the construction also , draw a triangle ABC with side BC = 6 cm, AB=5 cm and \(\angle \mathrm{ABC}\)=\(60^{\circ}\) . Then construct a triangle whose sides are \(\frac{3}{4}\) of the corresponding sides of the triangle ABC.

Ans : A \(\triangle \mathrm{A}^{\prime} \mathrm{BC}^{\prime}\) whose sides \(\frac{3}{4}\) of the corresponding sides of can be drawn as follows.
Step 1 Draw a \(\triangle\)ABC with side BC = 6 crn, AB = 5 cm and \(\angle\)ABC = 60\(^\circ\). 
Step 2 Draw a ray BX making an acute angle with BC on the opposite side of vertex A. Step 3 Locate 4 points (as 4 is greater in 3 and 4), \(B_{1}, B_{2}, B_{3}, B_{4}\), on line segment BX. 
Step 4 Join \(B_{4} C\)and draw a line through \(B_{3}\) , parallel to \(\mathrm{B}_{4} \mathrm{C}\) intersecting BC at \(C^{\prime}\) 
Step 5 Draw a line through C' parallel to AC intersecting AB at A'.\(\triangle \mathrm{A}^{\prime} \mathrm{BC}^{\prime}\) is the required triangle.

Justification
The construction can be justified by proving that, 
\(\mathrm{AB}=\frac{3}{4} \mathrm{AB}, \mathrm{BC}^{\prime}=\frac{3}{4} \mathrm{BC}, \mathrm{AC}^{\prime}=\frac{3}{4} \mathrm{AC}\)
\(\begin{array}{l}{\text { In } \triangle A^{\prime} B C^{\prime} \text { and } \triangle A B C \text { , }} \\ {\angle A^{\prime} C^{\prime} B=\angle A C B \text { (Corresponding angles) }} \\ {\angle A^{\prime} B C^{\prime}=\angle A B C(\text { Common})}\end{array}\)
\(\therefore \triangle A^{\prime} B C^{\prime} \sim \triangle A B C(\text { AA similarity criterion })\)
\(\Rightarrow \frac{A B}{A B}=\frac{B C}{B C}=\frac{A C}{A C}\)
\(\begin{array}{l}{\text { In } \triangle B B_{3} C^{\prime} \text { and } \triangle B B_{4} C} \\ {\angle B_{3} B C^{\prime}=\angle B_{4} B C(\text {Common})}\end{array}\)
\(\begin{array}{l}{\angle B B_{3} C^{\prime}=\angle B B_{4} C(\text { Corresponding angles) }} \\ {\therefore \triangle B B_{3} C^{\prime} \sim \Delta B B_{4} C(\text { AA similarity criterion) }}\end{array}\)
\(\begin{array}{l}{\Rightarrow \frac{\mathrm{BC}^{\prime}}{\mathrm{BC}}=\frac{\mathrm{BB}_{3}}{\mathrm{BB}_{4}}} \\ {\Rightarrow \frac{\mathrm{BC}^{\prime}}{\mathrm{BC}}=\frac{3}{4}} \\ {\text { From equations }(1) \text { and }(2), \text { we obtain }}\end{array}\)
\(\begin{array}{l}{\frac{A B}{A B}=\frac{B C}{B C}=\frac{A^{\prime} C^{\prime}}{A C}=\frac{3}{4}} \\ {\Rightarrow A B=\frac{3}{4} A B, B C^{\prime}=\frac{3}{4} B C, A C^{\prime}=\frac{3}{4} A C} \\ {\text { This justifies the construction. }}\end{array}\) 

NCERT / CBSE Book for Class 10 Maths

You can download the NCERT Book for Class 10 Maths in PDF format for free. Otherwise you can also buy it easily online.

• Click here for NCERT Book for Class 10 Maths
• Click here to buy NCERT Book for Class 10 Maths

All NCERT Solutions Class 10

• NCERT Solutions for Class 10 English
• NCERT Solutions for Class 10 Hindi
• NCERT Solutions for Class 10 Maths
• NCERT Solutions for Class 10 Science
• NCERT Solutions for Class 10 Social Science
• NCERT Solutions for Class 10 Sanskrit

All NCERT Solutions

You can also check out NCERT Solutions of other classes here. Click on the class number below to go to relevant NCERT Solutions of Class 1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12.

Class 4	Class 5	Class 6
Class 7	Class 8	Class 9
Class 10	Class 11	Class 12

Download the NCERT Solutions app for quick access to NCERT Solutions Class 10 Maths Chapter 11 Constructions. It will help you stay updated with relevant study material to help you top your class!

Previous Next

The post NCERT Solutions for Class 10 Maths Chapter 11 Constructions appeared first on AglaSem Schools.

from AglaSem Schools https://ift.tt/3dZuBec
https://ift.tt/2ScyeVT https://ift.tt/2ScyeVT