NCERT Solutions Class 11 Physics Chapter 7 System of Particles and Rotational Motion– Here are all the NCERT solutions for Class 11 Physics Chapter 7. This solution contains questions, answers, images, explanations of the complete chapter 7 titled OfSystem of particles and Rotational Motion taught in Class 11. If you are a student of Class 11 who is using NCERT Textbook to study Physics, then you must come across chapter 7 System of particles and Rotational Motion After you have studied the lesson, you must be looking for answers of its questions. Here you can get complete NCERT Solutions for Class 11 Physics Chapter 7 System of Particles and Rotational Motion in one place.
NCERT Solutions Class 11 Physics Chapter 7 System of particles and Rotational Motion
Here on AglaSem Schools, you can access to NCERT Book Solutions in free pdf for Physics for Class 11 so that you can refer them as and when required. The NCERT Solutions to the questions after every unit of NCERT textbooks aimed at helping students solving difficult questions.
For a better understanding of this chapter, you should also see summary of Chapter 7 System of particles and Rotational Motion , Physics, Class 11.
| Class | 11 |
| Subject | Physics |
| Book | Physics Part I |
| Chapter Number | 7 |
| Chapter Name |
System of particles and Rotational Motion |
NCERT Solutions Class 11 Physics chapter 7 System of particles and Rotational Motion
Class 11, Physics chapter 7, System of particles and Rotational Motion solutions are given below in PDF format. You can view them online or download PDF file for future use.
System of particles and Rotational Motion Download
Did you find NCERT Solutions Class 11 Physics chapter 7 System of particles and Rotational Motion helpful? If yes, please comment below. Also please like, and share it with your friends!
NCERT Solutions Class 11 Physics chapter 7 System of particles and Rotational Motion- Video
You can also watch the video solutions of NCERT Class11 Physics chapter 7 System of particles and Rotational Motion here.
Video – will be available soon.
If you liked the video, please subscribe to our YouTube channel so that you can get more such interesting and useful study resources.
Download NCERT Solutions Class 11 Physics chapter 7 System of particles and Rotational Motion In PDF Format
You can also download here the NCERT Solutions Class 11 Physics chapter 7 System of particles and Rotational Motion in PDF format.
Click Here to download NCERT Solutions for Class 11 Physics chapter 7 System of particles and Rotational Motion
Question & Answer
Q.1: Give the location of the centre of mass of a (i) sphere, (ii) cylinder. (iii) ring, and (iv) cube, each of uniform mass density. Does the centre of mass of a body necessarily lie inside the body?
Ans : Geometric centre; No The centre of mass (C.M.) is a point where the mass of a body is supposed to be concentrated. For the given geometric shapes having a uniform mass density, the C.M. lies at their respective geometric centres. The centre of mass of a body need not necessarily lie within it. For example, the C,M, of bodies such as a ring, a hollow sphere, etc., lies outside the body.
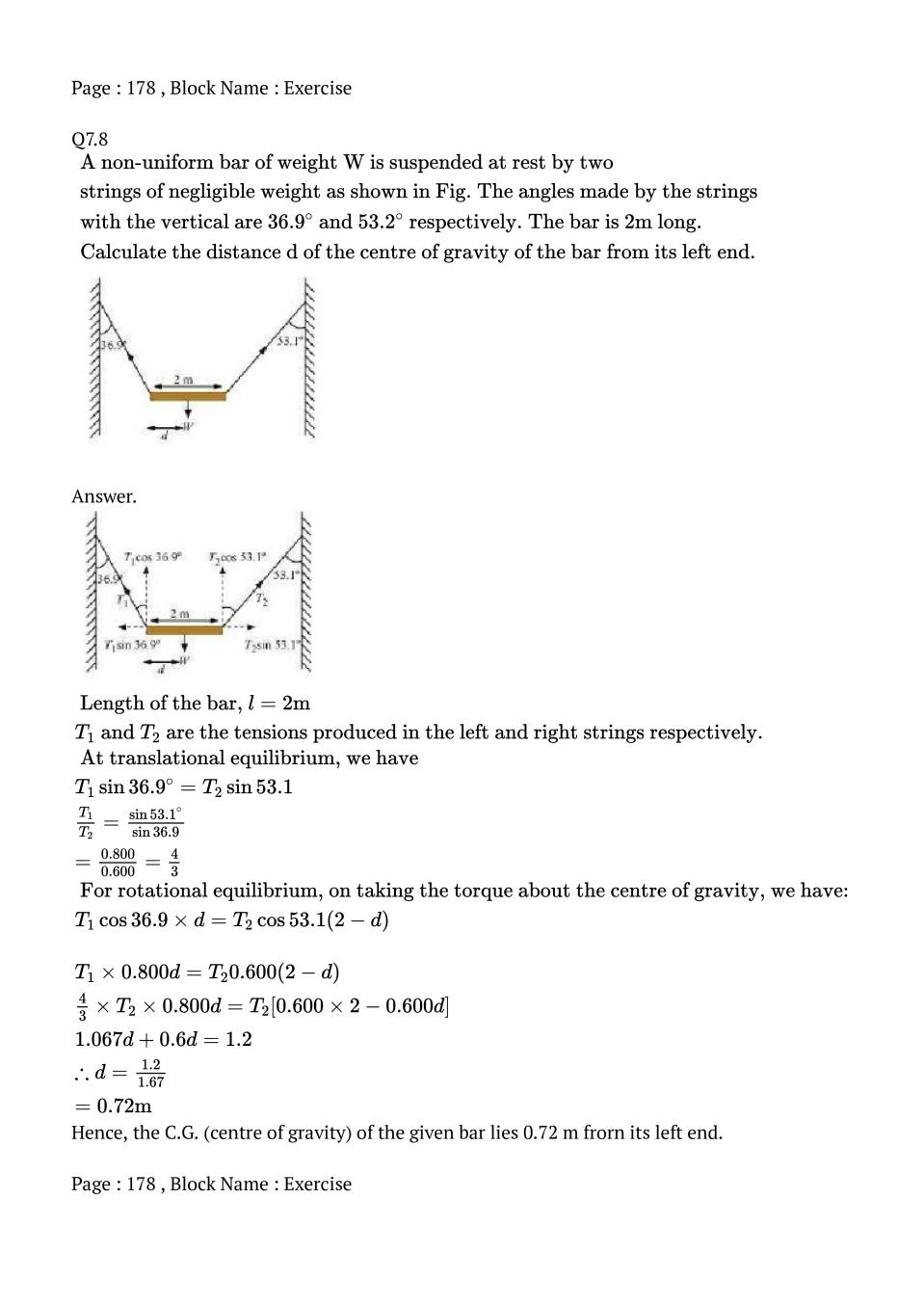
Q.2: child sits stationary at one end of a long trolley moving uniformly with a speed V on a smooth horizontal floor. If the child gets up and runs about on the trolley in any manner, what is the speed of the CM of the (trolley + child) system?
Ans : No change The child is running arbitrarily on a trolley moving with velocity v. However, the running of the child will produce no effect on the velocity of the centre of mass of the trolley. This is because the force due to the boy's motion is purely internal. Internal forces produce no effect on the moti«, cf the bodies on which they act. Since no external force is involved in the boy—trolley system, the boy's motion Hill produce no change in the velocity of the centre of mass of the trolley.
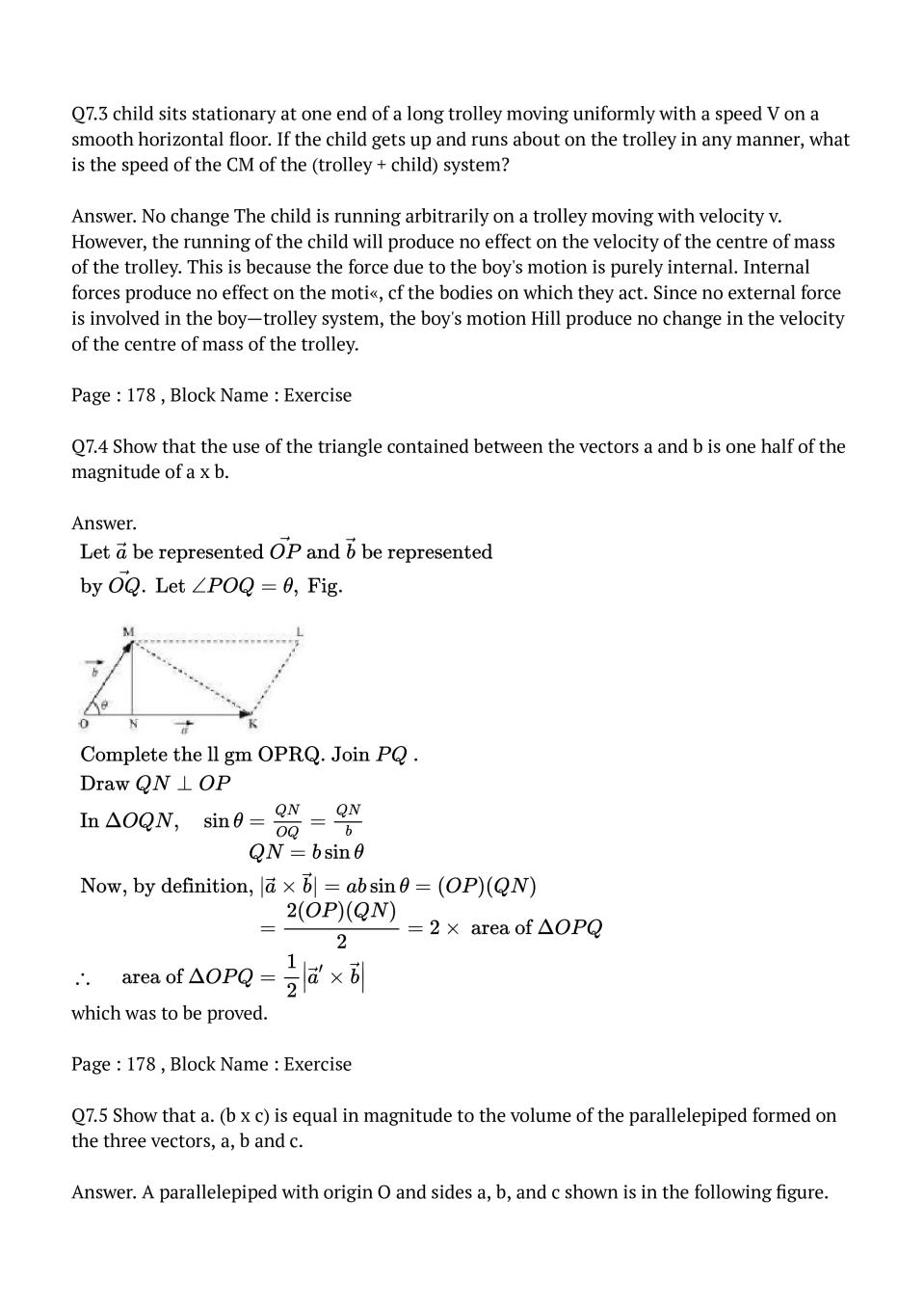
Q.3: Show that the use of the triangle contained between the vectors a and b is one half of the magnitude of a x b.
Ans : \(\begin{array}{l}{\text { Let } \vec{a} \text { be represented } \vec{O P} \text { and } \vec{b} \text { be represented }} \\ {\text { by } \vec{O Q} . \text { Let } \angle P O Q=\theta, \text { Fig. }}\end{array}\)\(\begin{array}{l}{\text { Complete the ll gm OPRQ. Join } P Q \text { . }} \\ {\text { Draw } Q N \perp O P} \\ {\text { In } \Delta O Q N, \quad \sin \theta=\frac{Q N}{O Q}=\frac{Q N}{b}}\end{array}\) \(\begin{array}{c}{Q N=b \sin \theta} \\ {\text { Now, by definition, }|\vec{a} \times \vec{b}|=a b \sin \theta=(O P)(Q N)}\end{array}\) \(\begin{aligned} &=\frac{2(O P)(Q N)}{2}=2 \times \text { area of } \Delta O P Q \\ \therefore \quad \text { area of } \Delta O P Q &=\frac{1}{2}\left|\vec{a}^{\prime} \times \vec{b}\right| \end{aligned}\) which was to be proved.
Q.4: Show that a. (b x c) is equal in magnitude to the volume of the parallelepiped formed on the three vectors, a, b and c.
Ans : A parallelepiped with origin O and sides a, b, and c shown is in the following figure.\(\begin{array}{l}{\text { Volume of the given parallelepiped = abc }} \\ {\vec{\mathrm{OC}}=\vec{a}} \\ {\vec{\mathrm{OB}}=\vec{b}} \\ {\vec{\mathrm{OC}}=\vec{c}}\end{array}\) \(\begin{array}{l}{\text { Let } \hat{\mathbf{n}} \text { be a unit vector perpendicular to both } b \text { and } c . \text { Hence, } \hat{\mathbf{n}} \text { and } a \text { have the same }} \\ {\text { direction. }}\end{array}\) \(\begin{array}{l}{\therefore \vec{b} \times \vec{c}=b c \sin \theta \hat{\mathbf{n}}} \\ {=b c \sin 90^{\circ} \hat{\mathbf{n}}} \\ {=b c \hat{n}} \\ {\vec{a} \cdot(\vec{b} \times \vec{c})} \\ {=a \cdot(b c \hat{\mathbf{n}})}\end{array}\) \(\begin{aligned} & \vec{a} \cdot(\vec{b} \times \vec{c}) \\ &=a \cdot(b c \hat{\mathbf{n}}) \\ &=a b c \cos \theta \hat{\mathbf{n}} \\ &=a b c \cos 0^{\circ} \\ &=a b c \\ &=\text { Volume of the parallelepiped } \end{aligned}\)
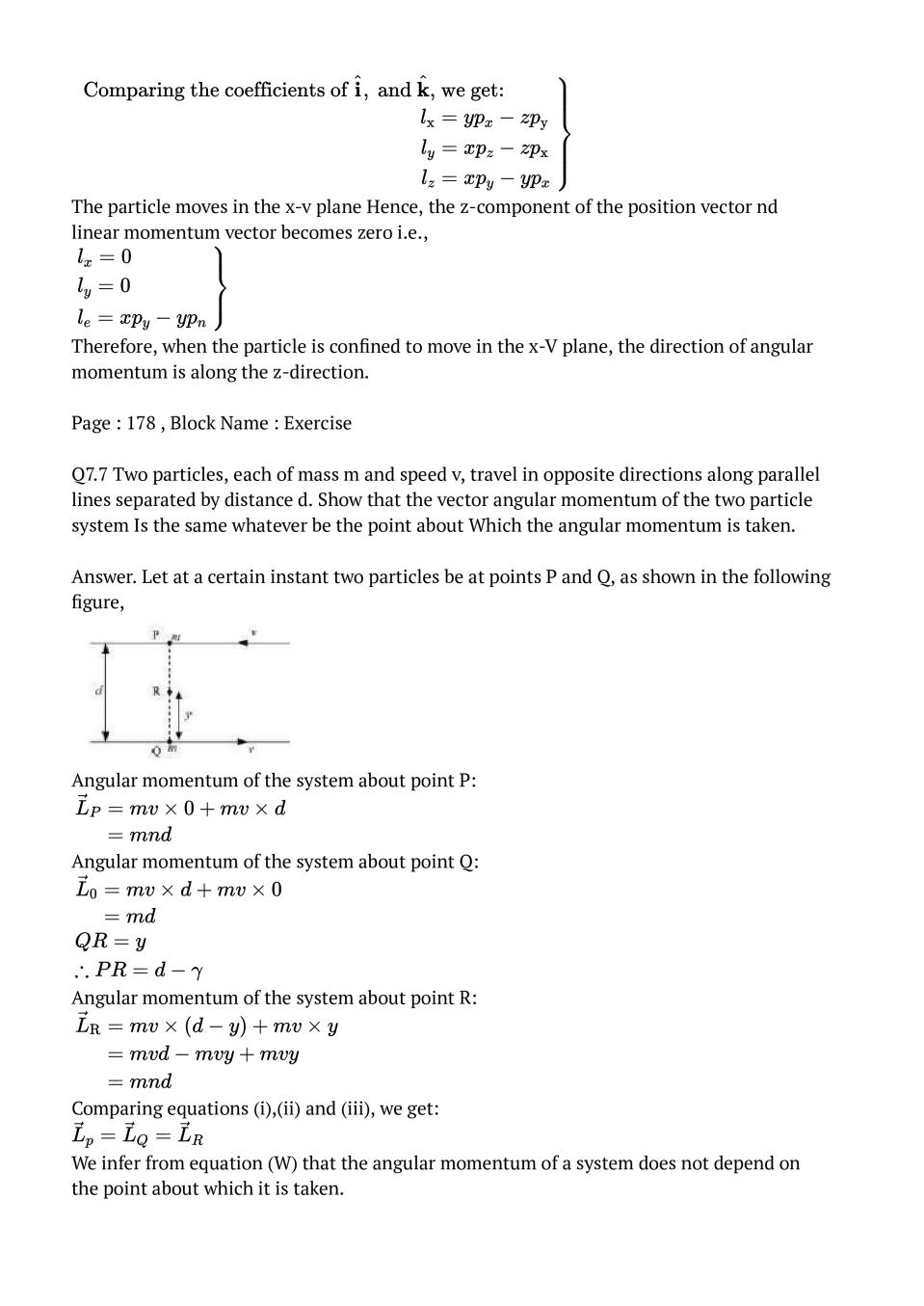
Q.5: Find the components along the x. y, z-axes of the angular momentum I of a particle, whose position vector is r with components x, y, z and momentum is p with components \(\mathrm{p}_{\mathrm{x}}, \mathrm{p}_{\mathrm{y}} \text { and } \mathrm{p}_{\mathrm{z}}\) Show that if the particle moves only in the x-y plane the angular momentum has only a z- component.
Ans : \(\begin{aligned} l_{x} &=y \rho_{z}-z \rho_{y} \\ l_{y} &=2 p_{x}-x p_{z} \\ l_{z} &=x p_{y}-y p_{x} \end{aligned}\) \(\begin{array}{l}{\text { Linear momentum of the particle, } \vec{p}=p_{x} \hat{\mathbf{i}}+p_{y} \mathbf{j}+p_{z} \hat{\mathbf{k}}} \\ {\text { Position vector of the particle, } \vec{r}=x \hat{\mathbf{i}}+y \hat{\mathbf{j}}+z \hat{\mathbf{k}}} \\ {\text { Angular momentum, } \vec{l}=\vec{r} \times \vec{p}}\end{array}\) \(=(x \hat{\mathbf{i}}+y \hat{\mathbf{j}}+z \hat{\mathbf{k}}) \times\left(p_{\mathbf{r}} \hat{\mathbf{i}}+p_{y} \hat{\mathbf{j}}+p_{r} \hat{\mathbf{k}}\right)\) \(\left.\begin{aligned} \text { Comparing the coefficients of } \hat{\mathbf{i}}, \text { and } \hat{\mathbf{k}}, & \text { we get: } \\ l_{\mathrm{x}} &=y p_{x}-z p_{\mathrm{y}} \\ l_{y} &=x p_{z}-z p_{\mathrm{x}} \\ l_{z} &=x p_{y}-y p_{x} \end{aligned}\right\}\) The particle moves in the x-v plane Hence, the z-component of the position vector nd linear momentum vector becomes zero i.e., \(\left.\begin{array}{l}{l_{x}=0} \\ {l_{y}=0} \\ {l_{e}=x p_{y}-y p_{n}}\end{array}\right\}\) Therefore, when the particle is confined to move in the x-V plane, the direction of angular momentum is along the z-direction.
NCERT / CBSE Book for Class 11 Physics
You can download the NCERT Book for Class 11 Physics in PDF format for free. Otherwise you can also buy it easily online.
- Click here for NCERT Book for Class 11 Physics
- Click here to buy NCERT Book for Class 11 Physics
All NCERT Solutions Class 11
- NCERT Solutions for Class 11 Accountancy
- NCERT Solutions for Class 11 Biology
- NCERT Solutions for Class 11 Chemistry
- NCERT Solutions for Class 11 Maths
- NCERT Solutions for Class 11 Economics
- NCERT Solutions for Class 11 History
- NCERT Solutions for Class 11 Geography
- NCERT Solutions for Class 11 Political Science
- NCERT Solutions for Class 11 Sociology
- NCERT Solutions for Class 11 Psychology
- NCERT Solutions for Class 11 English
- NCERT Solutions for Class 11 Hindi
- NCERT Solutions for Class 11 Physics
- NCERT Solutions for Class 11 Business Studies
- NCERT Solutions for Class 11 Statistics
All NCERT Solutions
You can also check out NCERT Solutions of other classes here. Click on the class number below to go to relevant NCERT Solutions of Class 1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12.
| Class 4 | Class 5 | Class 6 |
| Class 7 | Class 8 | Class 9 |
| Class 10 | Class 11 | Class 12 |
Download the NCERT Solutions app for quick access to NCERT Solutions Class 11 Physics Chapter 7 System of particles and Rotational Motion. It will help you stay updated with relevant study material to help you top your class!
The post NCERT Solutions for Class 11 Physics Chapter 7 System of particles and Rotational Motion appeared first on AglaSem Schools.
from AglaSem Schools https://ift.tt/3npsBPz
https://ift.tt/3tUM9h7 https://ift.tt/3tUM9h7










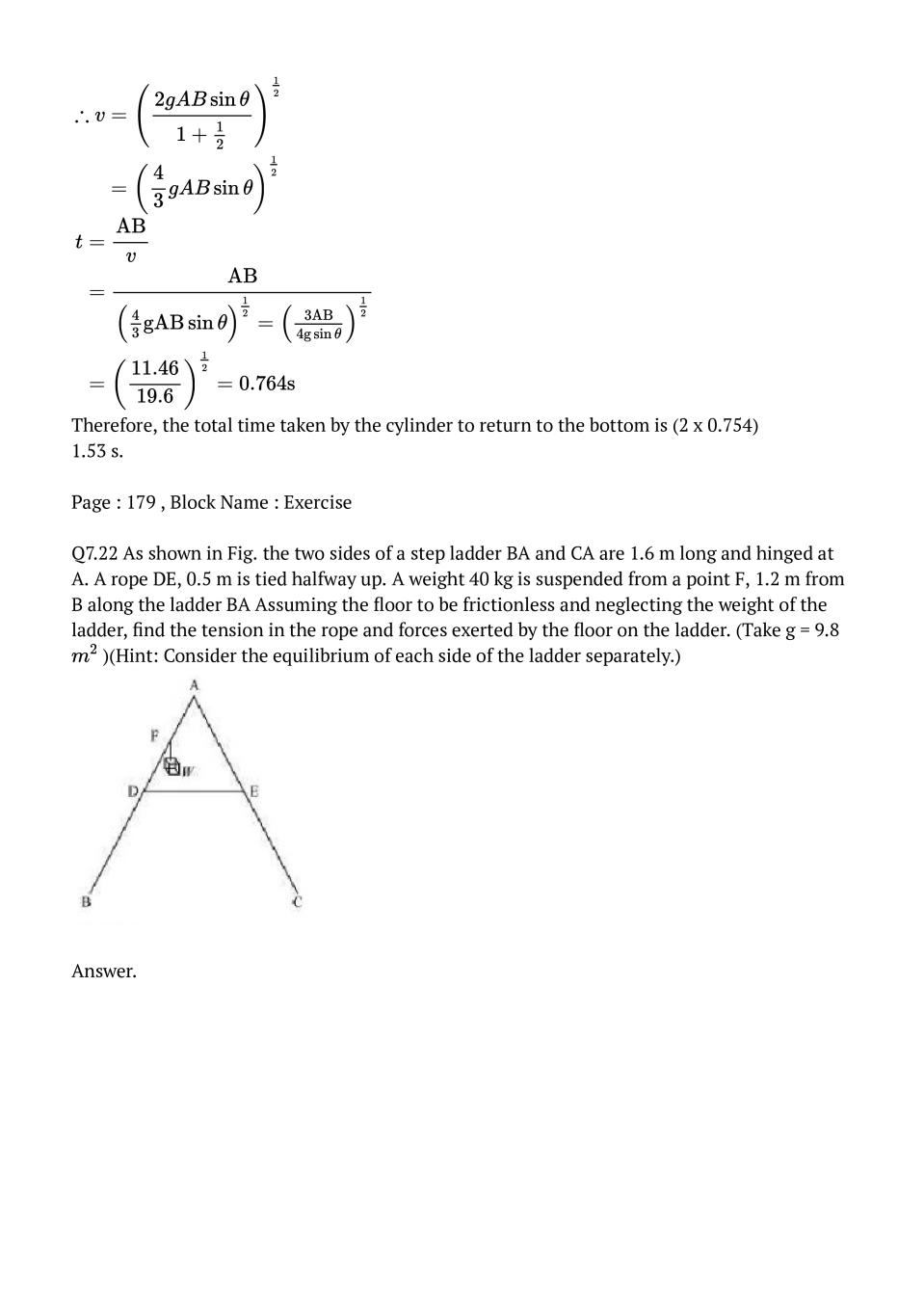
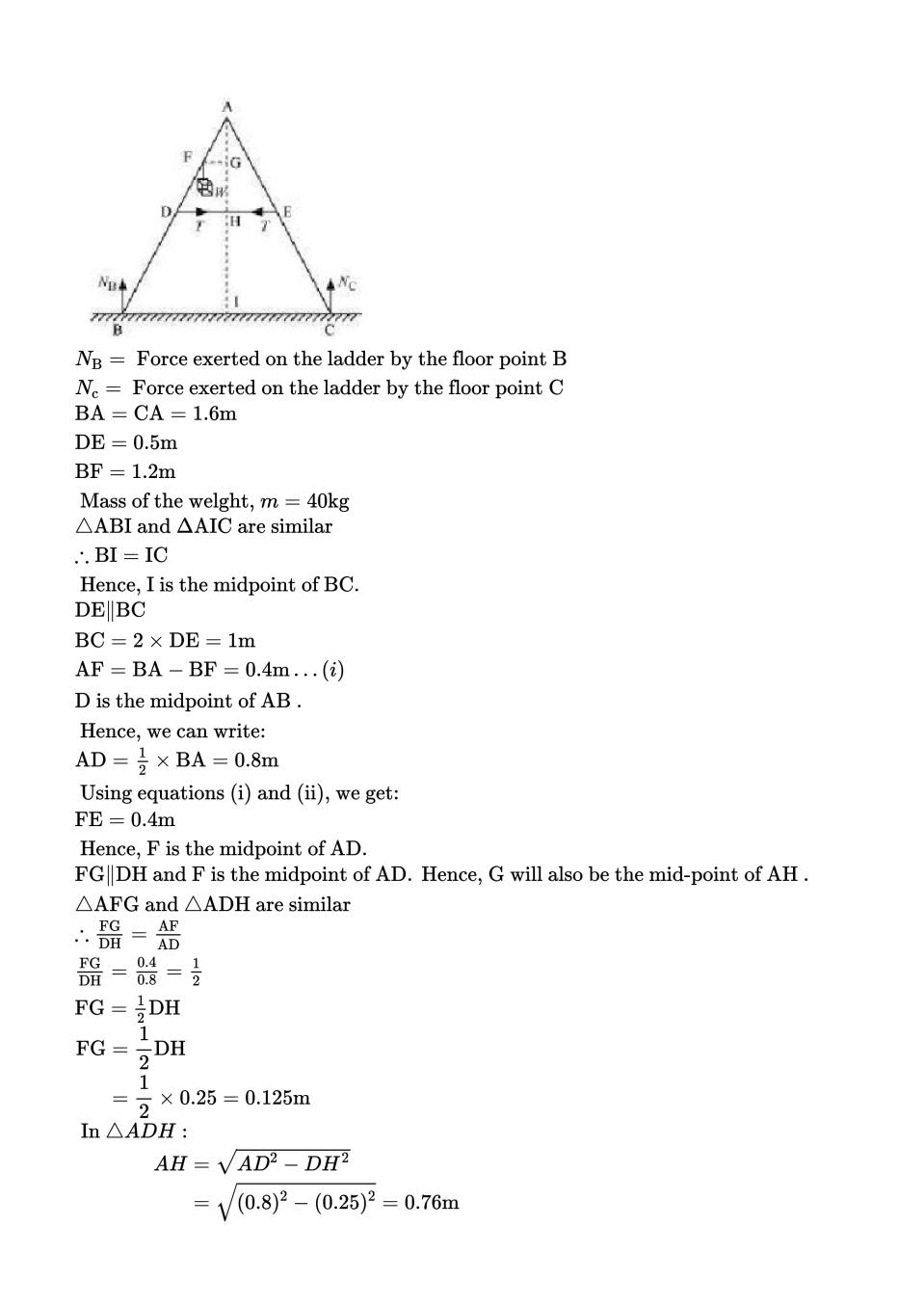






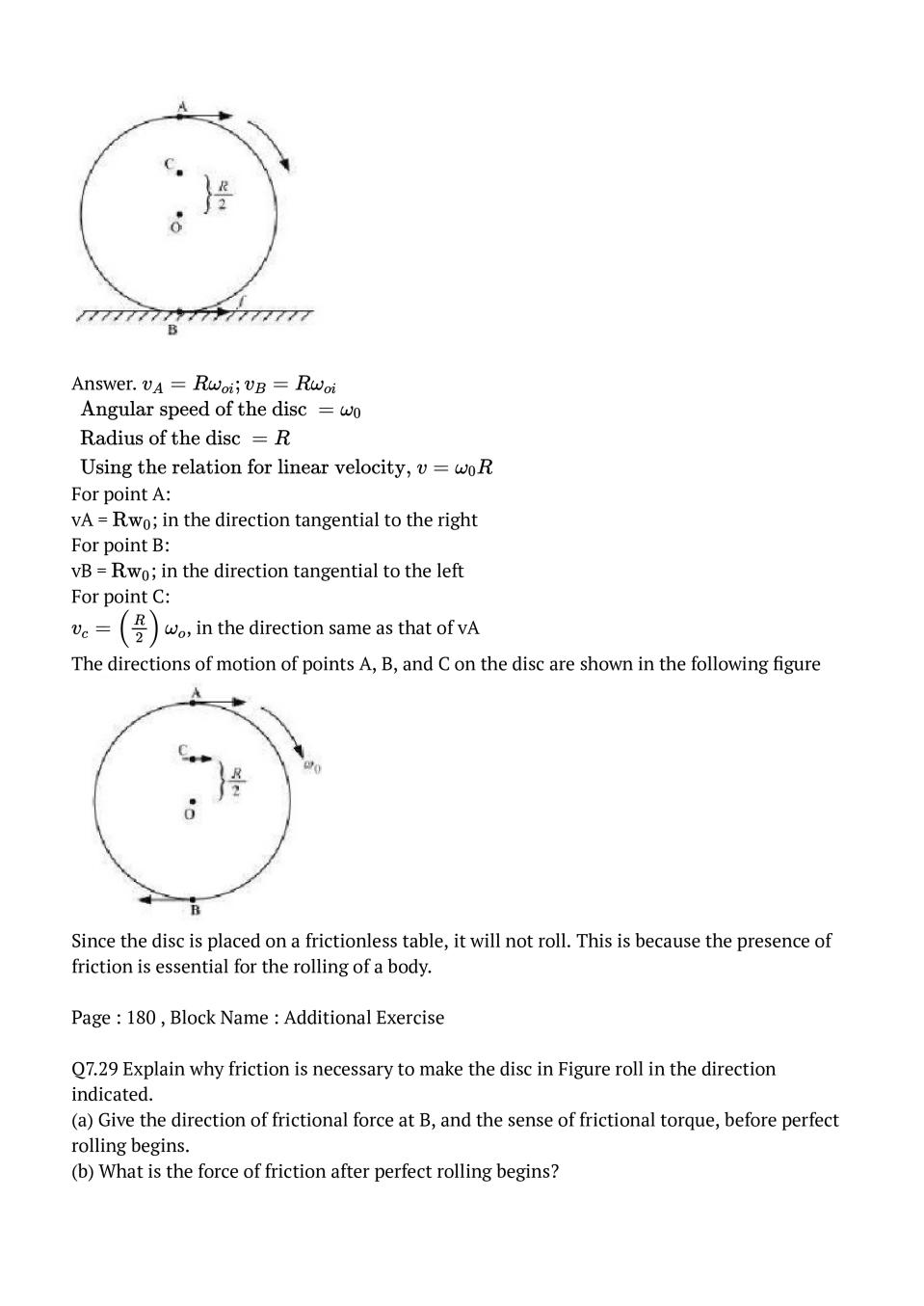






إرسال تعليق