Breaking News
Join telegram chanel https://t.me/GradeupEarning Make money online 100% legal

NCERT Solutions Class 11 Chemistry Chapter 5 States of Matter– Here are all the NCERT solutions for Class 11 Chemistry Chapter 5. This solution contains questions, answers, images, explanations of the complete chapter 1 titled States of Matter taught in Class 11. If you are a student of Class 11 who is using NCERT Textbook to study Chemistry, then you must come across chapter 5 States of Matter After you have studied the lesson, you must be looking for answers of its questions. Here you can get complete NCERT Solutions for Class 11 Chemistry Chapter 5 States of Matter in one place.

Go to Exercise

NCERT Solutions Class 11 Chemistry Chapter 5 States of Matter

Here on AglaSem Schools, you can access to NCERT Book Solutions in free pdf for Chemistry for Class 11 so that you can refer them as and when required. The NCERT Solutions to the questions after every unit of NCERT textbooks aimed at helping students solving difficult questions.

For a better understanding of this chapter, you should also see summary of Chapter 5 States of Matter , Chemistry, Class 11.

Class 11
Subject Chemistry
Book Chemistry Part I
Chapter Number 5
Chapter Name

States of Matter

NCERT Solutions Class 11 Chemistry chapter 5 States of Matter

Class 11, Chemistry chapter 5, States of Matter solutions are given below in PDF format. You can view them online or download PDF file for future use.

States of Matter Download

NCERT Solutions for Class 11 Chemistry Chapter 5 States of Matter & Solid State Download

Did you find NCERT Solutions Class 11 Chemistry chapter 5 States of Matter helpful? If yes, please comment below. Also please like, and share it with your friends!

NCERT Solutions Class 11 Chemistry chapter 5 States of Matter- Video

You can also watch the video solutions of NCERT Class11 Chemistry chapter 5 States of Matter here.

Video – will be available soon.

If you liked the video, please subscribe to our YouTube channel so that you can get more such interesting and useful study resources.

Download NCERT Solutions Class 11 Chemistry chapter 5 States of Matter In PDF Format

You can also download here the NCERT Solutions Class 11 Chemistry chapter 5 States of Matter in PDF format.

Click Here to download NCERT Solutions for Class 11 Chemistry chapter 5 States of Matter

Question & Answer

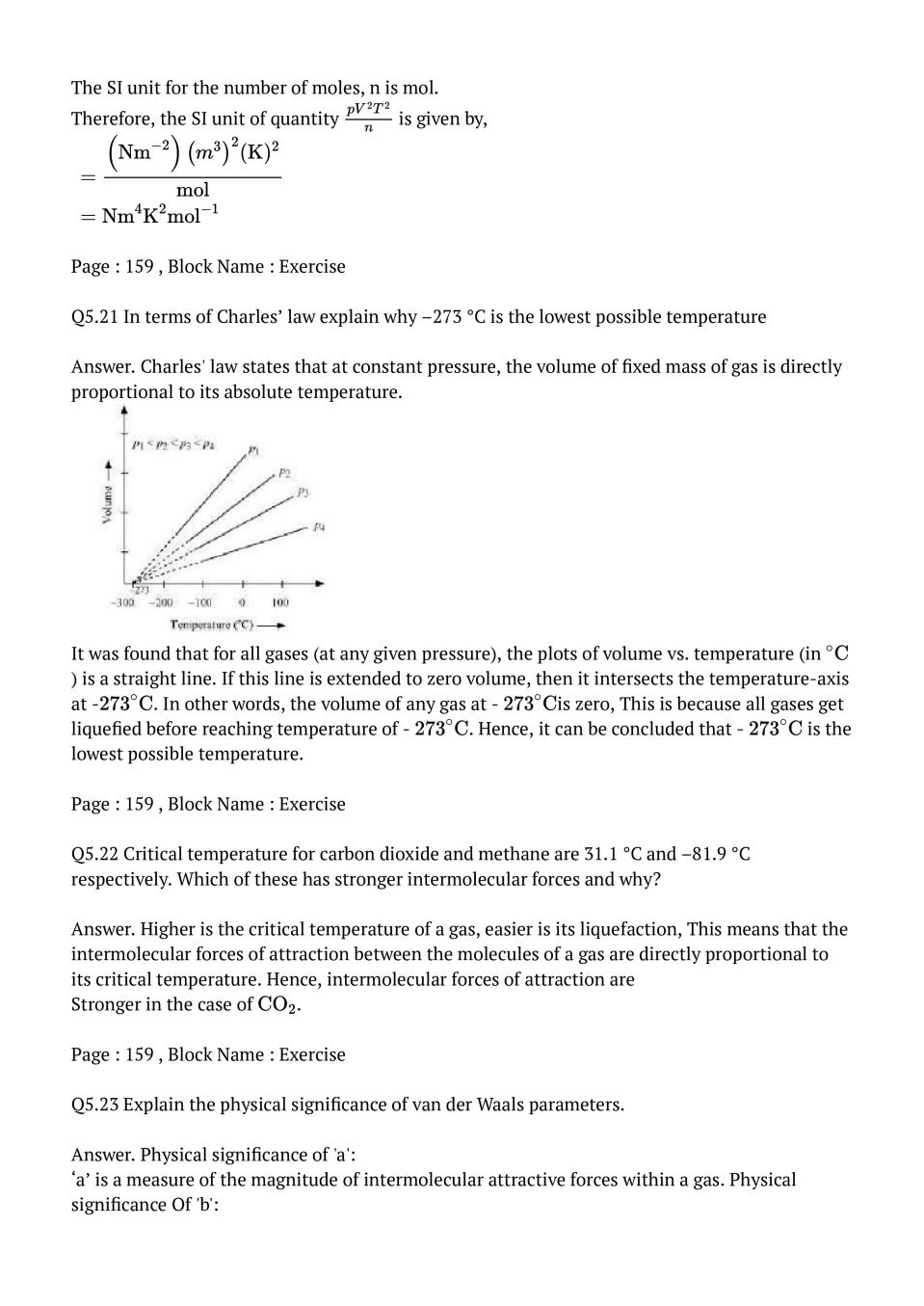
Q.1: What will be the minimum pressure required to compress 500 \(\mathrm{dm}^{3}\) of air at 1 bar to 200 \(\mathrm{dm}^{3}\) at 30°C?

Ans : Given, 
Initial pressure, \(P_{1}\) = 1 bar 
Initial volume, \(V_{1}\) = 500  \(\mathrm{dm}^{3}\)
Final volume, \(V_{2}\) = 200 \(\mathrm{dm}^{3}\) 
Since the temperature remains constant, the final pressure \((P_{2})\) can be calculated using Boyle's law. 
According to Boyle's law, 
\(\begin{aligned} p_{1} V_{1} &=p_{2} V_{2} \\ \Rightarrow p_{2} &=\frac{p_{1} V_{1}}{V_{2}} \\ &=\frac{1 \times 500}{200} \mathrm{bar} \\ &=2.5 \mathrm{bar} \end{aligned}\)
Therefore, the minimum pressure required is 2.5 bar. 
Q.2: A vessel of 120 mL capacity contains a certain amount of gas at 35 °C and 1.2 bar pressure. The gas is transferred to another vessel of volume 180 mL at 35 °C. What would be its pressure?

Ans : Given, 
Initial pressure, \(P_{1}\) = 1.2 bar 
Initial volume, \(V_{1}\) = 120 mL 
Final volume, \(V_{2}\) = 180 mL Since the temperature remains constant, the final pressure \((p_{2})\) can be calculated using Boyle's law 
According to Boyle's law,
\(\begin{aligned} p_{1} V_{1} &=p_{2} V_{2} \\ p_{2} &=\frac{p_{1} V_{1}}{V_{2}} \\ &=\frac{1.2 \times 120}{180} \text { bar } \\ &=0.80 \\ \text { Therefore, the pressure would be } 0.8 \text { bar. } \end{aligned}\) 
Q.3: Using the equation of state pV=nRT; show that at a given temperature density of a gas is proportional to gas pressure p.

Ans : The equation of state is given by, 
\(\rho V=n R T \ldots \ldots \ldots\)
Where, 
P \(\rightarrow\) Pressure of gas  
V \(\rightarrow\) Volume of gas 
n \(\rightarrow\) Number of moles of gas 
R \(\rightarrow\) Gas constant 
T \(\rightarrow\) Temperature of gas 
From equation (i) we have, 
\(\begin{array}{l}{\frac{n}{V}=\frac{p}{\mathrm{R} T}} \\ {\text { Replacing } n \text { with } \frac{m}{M}, \text { we have }} \\ {\frac{m}{M V}=\frac{p}{\mathrm{R} T} \ldots \ldots \ldots(\text { ii })} \\ {\text { Where, }} \\ {m \rightarrow \text { Mass of gas }} \\ {M \rightarrow \text { Molar mass of gas }}\end{array}\)
\(\begin{array}{l}{\frac{m}{V}=d} \\ {\text { Thus, from equation (ii), we have }} \\ {\frac{d}{M}=\frac{p}{\mathrm{R} T}} \\ {\Rightarrow d=\left(\frac{M}{\mathrm{R} T}\right) P}\end{array}\)
\(\begin{array}{l}{\text { Molar mass }(M) \text { of a gas is always constant and therefore, at constant temperature }} \\ {(T), \frac{M}{R T}=\text { constant. }} \\ {d=(\text { constant }) p} \\ {\Rightarrow d \propto p} \\ {\text { Hence, at a given temperature, the density (d) of gas is proportional to its pressure (p) }}\end{array}\) 
Q.4: At 0°C, the density of a certain oxide of a gas at 2 bar is same as that of dinitrogen at 5 bar. What is the molecular mass of the oxide?

Ans : \(\begin{array}{l}{\text { Density (d) of the substance at temperature (T) can be given by the expression, }} \\ {\quad d=\frac{M p}{R T}} \\ {\text { Now, density of oxide }\left(d_{1}\right) \text { is given by, }}\end{array}\)
\(\begin{array}{l}{d_{1}=\frac{M_{1} p_{1}}{R T}} \\ {\text { Where, } M_{2} \text { and } p_{1} \text { are the mass and pressure of the oxide respectively. }} \\ {\text { Density of dinitrogen gas }\left(d_{2}\right) \text { ls given by, }} \\ {d_{2}=\frac{M_{2} p_{2}}{R T}}\end{array}\)
\(\begin{array}{l}{\text { Where, } M_{2} \text { and } p_{2} \text { are the mass and pressure of the oxide respectively. }} \\ {\text { According to the given question, }} \\ {d_{1}=d_{2}} \\ {\therefore M_{1} p_{1}=M_{2} p_{2}} \\ {\text { Given, }} \\ {p_{1}=2 b a r} \\ {p_{2}=5 \text { bar }}\end{array}\)
\(\begin{aligned} \text { Molecular } & \text { mass of nitrogen, } M_{2}=28 \mathrm{g} / \mathrm{mol} \\ \mathrm{Now}, M_{1} &=\frac{M_{2} p_{2}}{p_{1}} \\ &=\frac{28 \times 5}{2} \\ &=70 \mathrm{g} / \mathrm{mol} \\ \text { Hence, the molecular mass of the oxlde is } 70 \mathrm{g} / \mathrm{mol} \end{aligned}\) 
Q.5: Pressure of 1 g of an ideal gas A at 27 °C is found to be 2 bar. When 2 g of another ideal gas B is introduced in the same flask at same temperature the pressure becomes 3 bar. Find a relationship between their molecular masses.

Ans : \(\begin{array}{l}{\text { For ideal gas } A, \text { the ideal gas equation is given by, }} \\ {p_{\mathrm{A}} V=n_{\mathrm{A}} \mathrm{RT} \ldots \ldots \text { (i) }}\end{array}\)
Where, \(p_{\mathrm{A}} \text { and } \mathrm{n}_{\mathrm{B}}\) represent the pressure and number of moles of gas A. 
For ideal gas B, the ideal gas equation is given by,
\(p_{B} V=n_{B} \mathrm{RT} \ldots \ldots .\)
Where, p and n, represent the pressure and number of moles of gas B. 
[ V and T are constants for gases A and B ] 
From equation (i), we have
\(p_{A} V=\frac{m_{A}}{\mathbf{M}_{A}} \mathrm{R} T \Rightarrow \frac{p_{A} \mathrm{M}_{A}}{m_{A}}=\frac{\mathrm{RT}}{V} \ldots \ldots \ldots\)
From equation (ii), we have 
\(p_{\mathrm{B}} V=\frac{m_{\mathrm{B}}}{\mathrm{M}_{\mathrm{B}}} \mathrm{R} T \Rightarrow \frac{p_{\mathrm{B}} \mathrm{M}_{\mathrm{B}}}{m_{\mathrm{B}}}=\frac{\mathrm{R} T}{V} \ldots \ldots \ldots\)
Where, \(\mathrm{M}_{\mathrm{A}} \text { and } \mathrm{M}_{\mathrm{B}}\) are the molecular masses of gases A and B respectively. 
Now, from equations (iii) and (iv) we have 
\(\frac{p_{A} \mathbf{M}_{A}}{m_{A}}=\frac{p_{g} \mathbf{M}_{B}}{m_{B}} \ldots \ldots \ldots(v)\)
Given, 
\(\begin{array}{l}{m_{A}=1 \mathrm{g}} \\ {p_{A}=2 \text { bar }} \\ {m_{B}=2 \mathrm{g}} \\ {p_{B}=(3-2)=1 \mathrm{bar}}\end{array}\)
\(\begin{array}{l}{\text { (Since total pressure is } 3 \text { bar) }} \\ {\text { Substituting these values in equation (v), we have }} \\ {\frac{2 \times \mathrm{M}_{A}}{1}=\frac{1 \times \mathrm{M}_{B}}{2}} \\ {\Rightarrow4\mathrm{M}_{A}=\mathrm{M}_{B}}\end{array}\)
\(\begin{array}{l}{\text { Thus, a relationship between the molecular masses of } A \text { and } B \text { is given by }} \\ {4 M_{A}=M_{B}}\end{array}\)

NCERT / CBSE Book for Class 11 Chemistry

You can download the NCERT Book for Class 11 Chemistry in PDF format for free. Otherwise you can also buy it easily online.

  • Click here for NCERT Book for Class 11 Chemistry
  • Click here to buy NCERT Book for Class 11 Chemistry

All NCERT Solutions Class 11

All NCERT Solutions

You can also check out NCERT Solutions of other classes here. Click on the class number below to go to relevant NCERT Solutions of Class 1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12.

Class 4 Class 5 Class 6
Class 7 Class 8 Class 9
Class 10 Class 11 Class 12

Download the NCERT Solutions app for quick access to NCERT Solutions Class 11 Chemistry Chapter 5 States of Matter. It will help you stay updated with relevant study material to help you top your class!

Previous  Next

The post NCERT Solutions for Class 11 Chemistry Chapter 5 States of Matter appeared first on AglaSem Schools.



from AglaSem Schools https://ift.tt/3xsSQJJ
https://ift.tt/eA8V8J https://ift.tt/eA8V8J
Tags

Post a Comment

إرسال تعليق

أحدث أقدم