NCERT Solutions Class 10 Maths Chapter 9 Some Applications of Trigonometry – Here are all the NCERT solutions for Class 10 Maths Chapter 9. This solution contains questions, answers, images, explanations of the complete Chapter 9 titled Some Applications of Trigonometry of Maths taught in Class 10. If you are a student of Class 10 who is using NCERT Textbook to study Maths, then you must come across Chapter 9 Some Applications of Trigonometry. After you have studied lesson, you must be looking for answers of its questions. Here you can get complete NCERT Solutions for Class 10 Maths Chapter 9 Some Applications of Trigonometry in one place.
NCERT Solutions Class 10 Maths Chapter 9 Some Applications of Trigonometry
Here on AglaSem Schools, you can access to NCERT Book Solutions in free pdf for Maths for Class 10 so that you can refer them as and when required. The NCERT Solutions to the questions after every unit of NCERT textbooks aimed at helping students solving difficult questions.
For a better understanding of this chapter, you should also see summary of Chapter 9 Some Applications of Trigonometry , Maths, Class 10.
| Class | 10 |
| Subject | Maths |
| Book | Mathematics |
| Chapter Number | 9 |
| Chapter Name |
Some Applications of Trigonometry |
NCERT Solutions Class 10 Maths chapter 9 Some Applications of Trigonometry
Class 10, Maths chapter 9, Some Applications of Trigonometry solutions are given below in PDF format. You can view them online or download PDF file for future use.
Some Applications of Trigonometry Download
Did you find NCERT Solutions Class 10 Maths chapter 9 Some Applications of Trigonometry helpful? If yes, please comment below. Also please like, and share it with your friends!
NCERT Solutions Class 10 Maths chapter 9 Some Applications of Trigonometry- Video
You can also watch the video solutions of NCERT Class10 Maths chapter 9 Some Applications of Trigonometry here.
If you liked the video, please subscribe to our YouTube channel so that you can get more such interesting and useful study resources.
Download NCERT Solutions Class 10 Maths chapter 9 Some Applications of Trigonometry In PDF Format
You can also download here the NCERT Solutions Class 10 Maths chapter 9 Some Applications of Trigonometry in PDF format.
Click Here to download NCERT Solutions for Class 10 Maths chapter 9 Some Applications of Trigonometry
Question & Answer
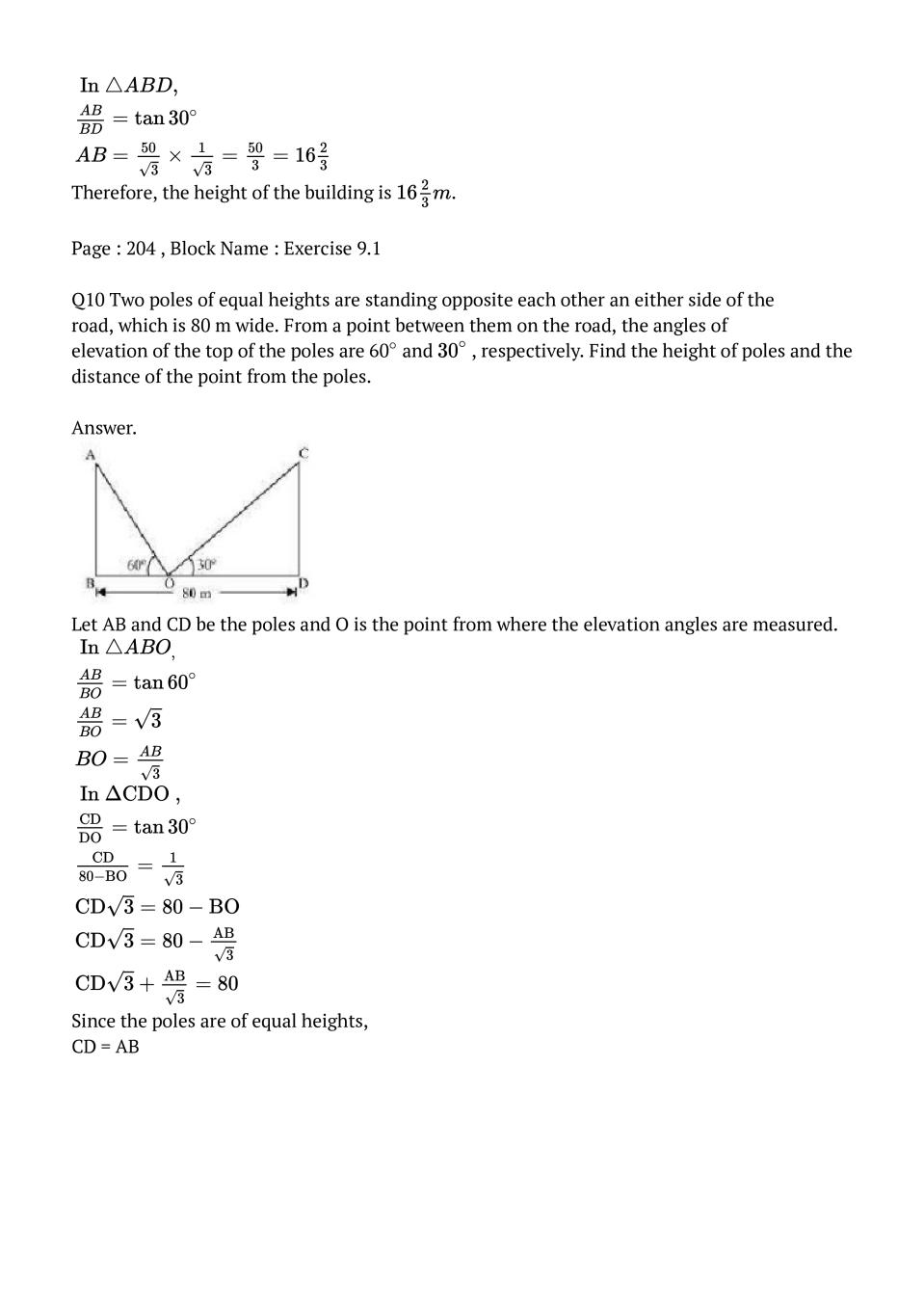
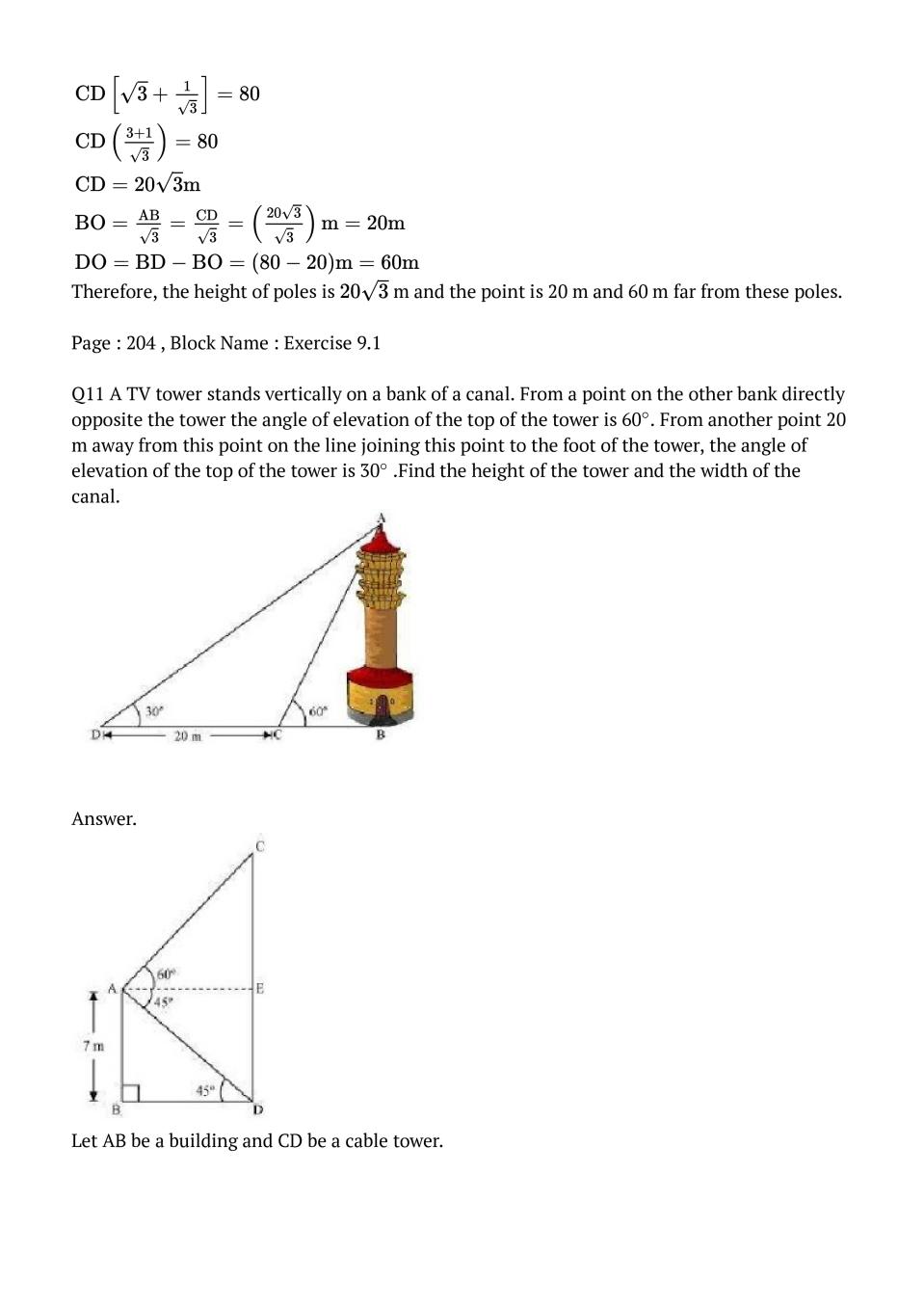
Q.1: A circus artist is climbing a 20 m long rope, which is tightly stretched and tied from the top of a vertical pole to the ground. Find the height of the pole, if the angle made by the rope with the ground level is \(30^{\circ}.\)
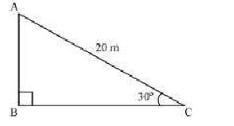
Ans : \( \begin{array}{l} \text{It can be observed from the figure that AB is the pole.}{\text { In } \triangle A B C,} \\ {\frac{A B}{A C}=\sin 30^{\circ}} \\ {\frac{A B}{20}=\frac{1}{2}} \\ {A B=\frac{20}{2}=10} \\ {\text { Therefore, the height of the pole is } 10 \mathrm{m} \text { . }}\end{array}\)
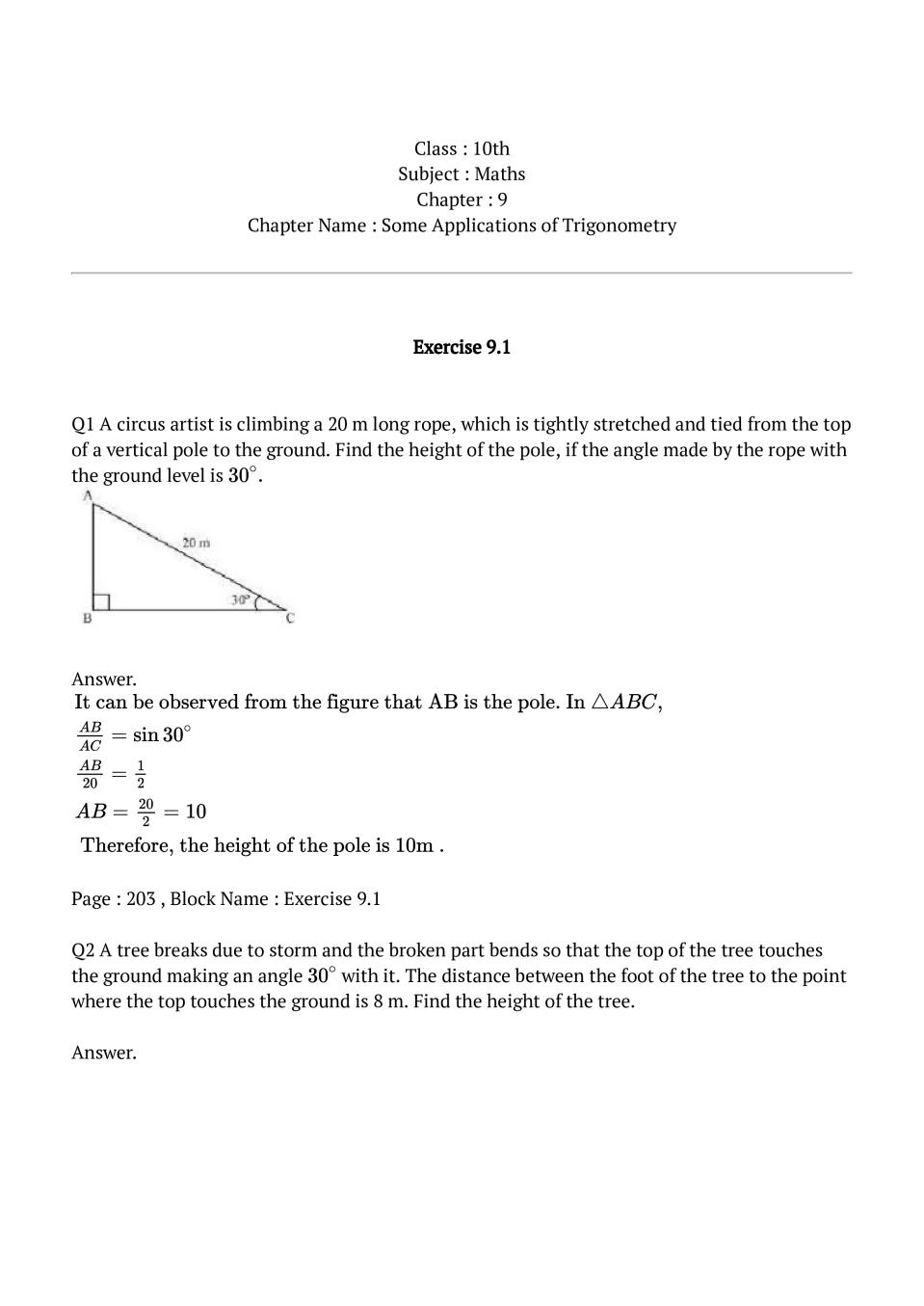
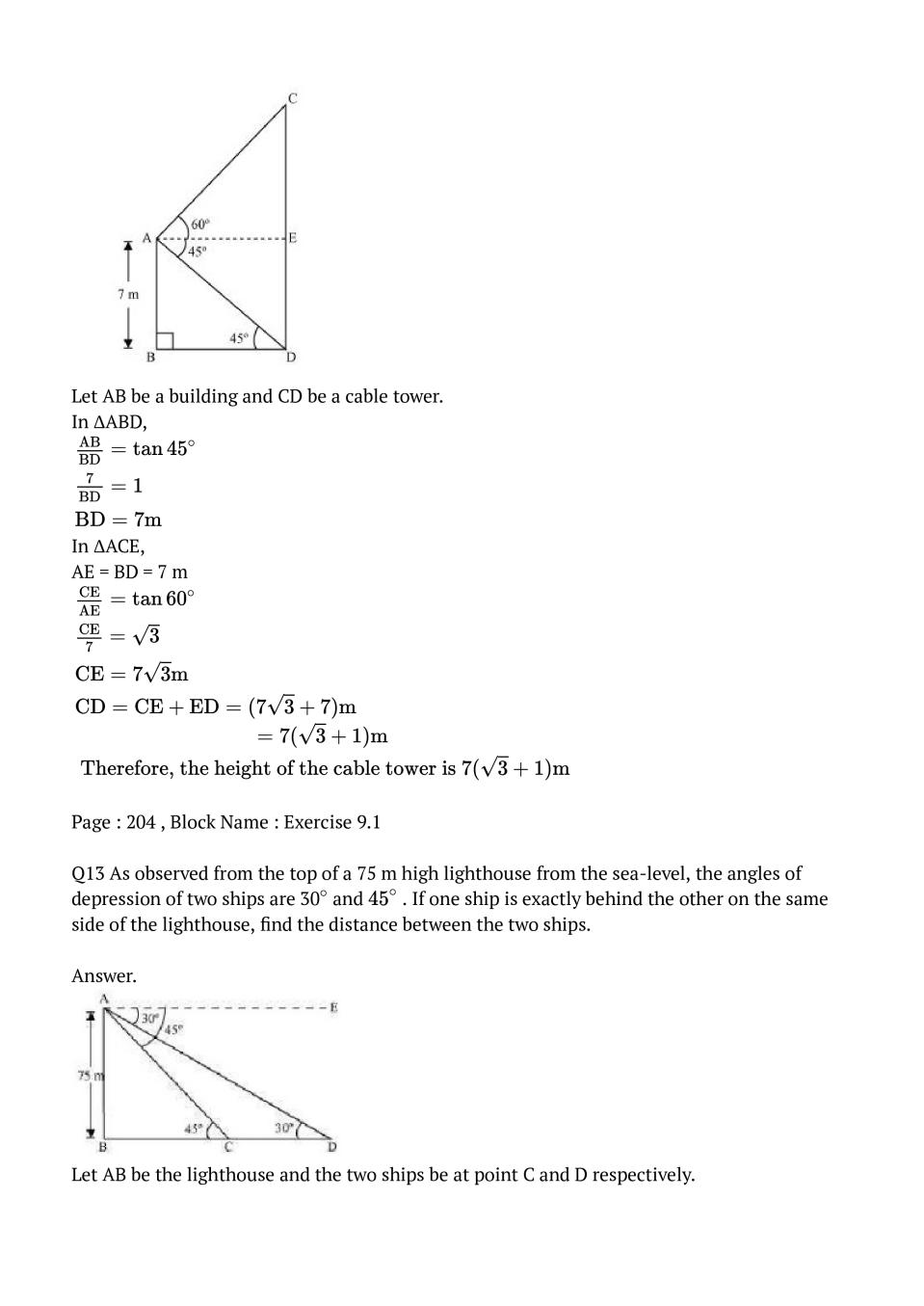
Q.2: A tree breaks due to storm and the broken part bends so that the top of the tree touches the ground making an angle \(30^{\circ}\) with it. The distance between the foot of the tree to the point where the top touches the ground is 8 m. Find the height of the tree.
Ans :Let AC was the original tree. Due to storm, it was broken into parts. The broken part \(\mathrm{A}^{\prime} \mathrm{B} \text { is making } 30^{\circ}\) with the ground. \(\begin{array}{l}{\text { In } \Delta \mathrm{A}^{\prime} \mathrm{BC}} \\ {\frac{\mathrm{BC}}{\mathrm{A}^{\prime} \mathrm{C}}=\tan 30^{\circ}} \\ {\frac{\mathrm{BC}}{8}=\frac{1}{\sqrt{3}}} \\ {\mathrm{BC}=\left(\frac{8}{\sqrt{3}}\right) \mathrm{m}}\end{array}\) \(\begin{array}{l}{\frac{\mathrm{A}^{\prime} \mathrm{C}}{\mathrm{A}^{\prime} \mathrm{B}}=\cos 30^{\circ}} \\ {\frac{8}{\mathrm{A}^{\prime} \mathrm{B}}=\frac{\sqrt{3}}{2}} \\ {\mathrm{A}^{\prime} \mathrm{B}=\left(\frac{16}{\sqrt{3}}\right) \mathrm{m}} \\ {\text { Height of tree }=\mathrm{A}^{\prime} \mathrm{B}+\mathrm{BC}}\end{array}\) \(\begin{aligned} &=\left(\frac{16}{\sqrt{3}}+\frac{8}{\sqrt{3}}\right) \mathrm{m}=\frac{24}{\sqrt{3}} \mathrm{m} \\ &=8 \sqrt{3} \mathrm{m} \end{aligned}\) Hence, the height of the tree is \(8 \sqrt{3} \mathrm{m}\).
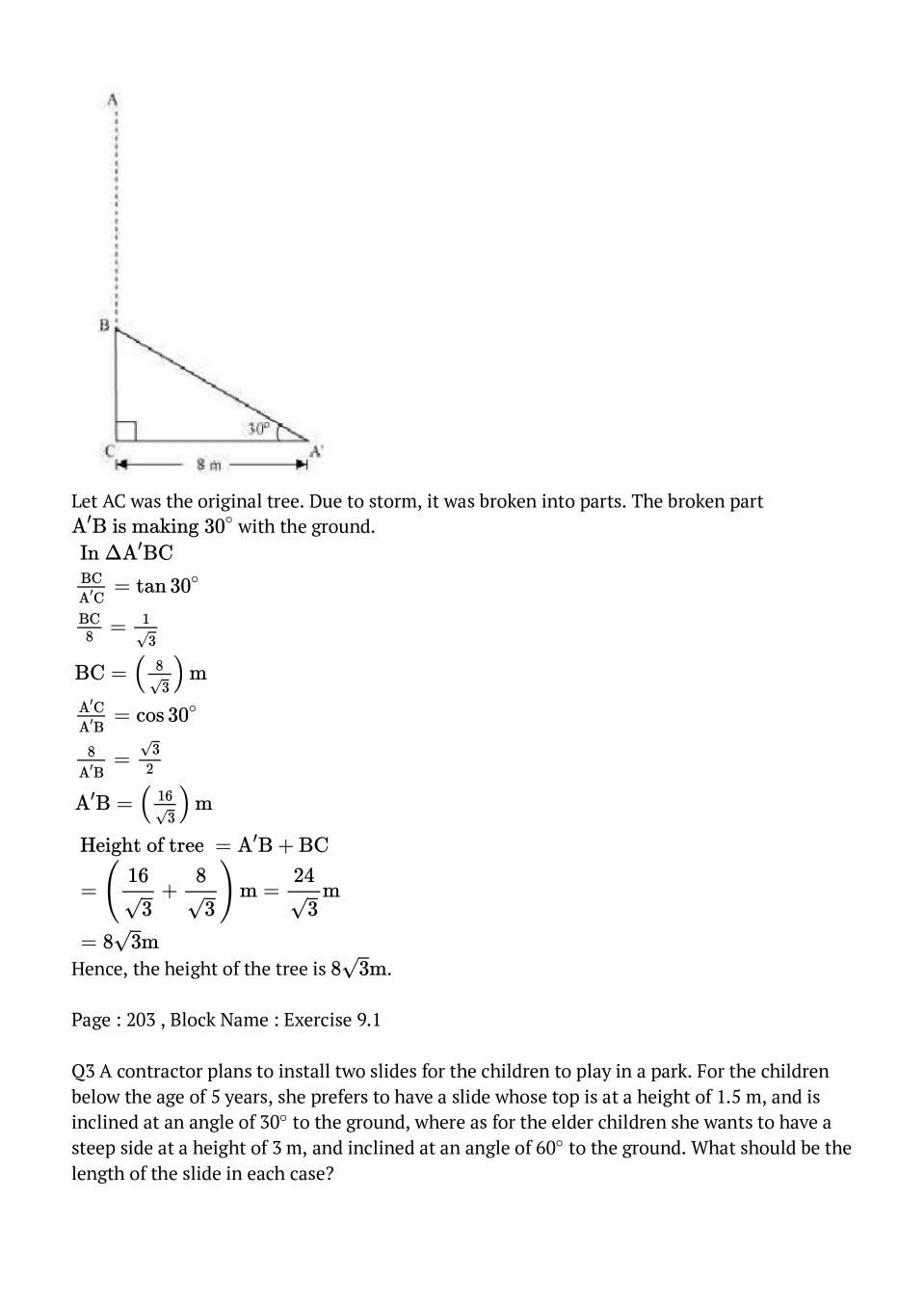
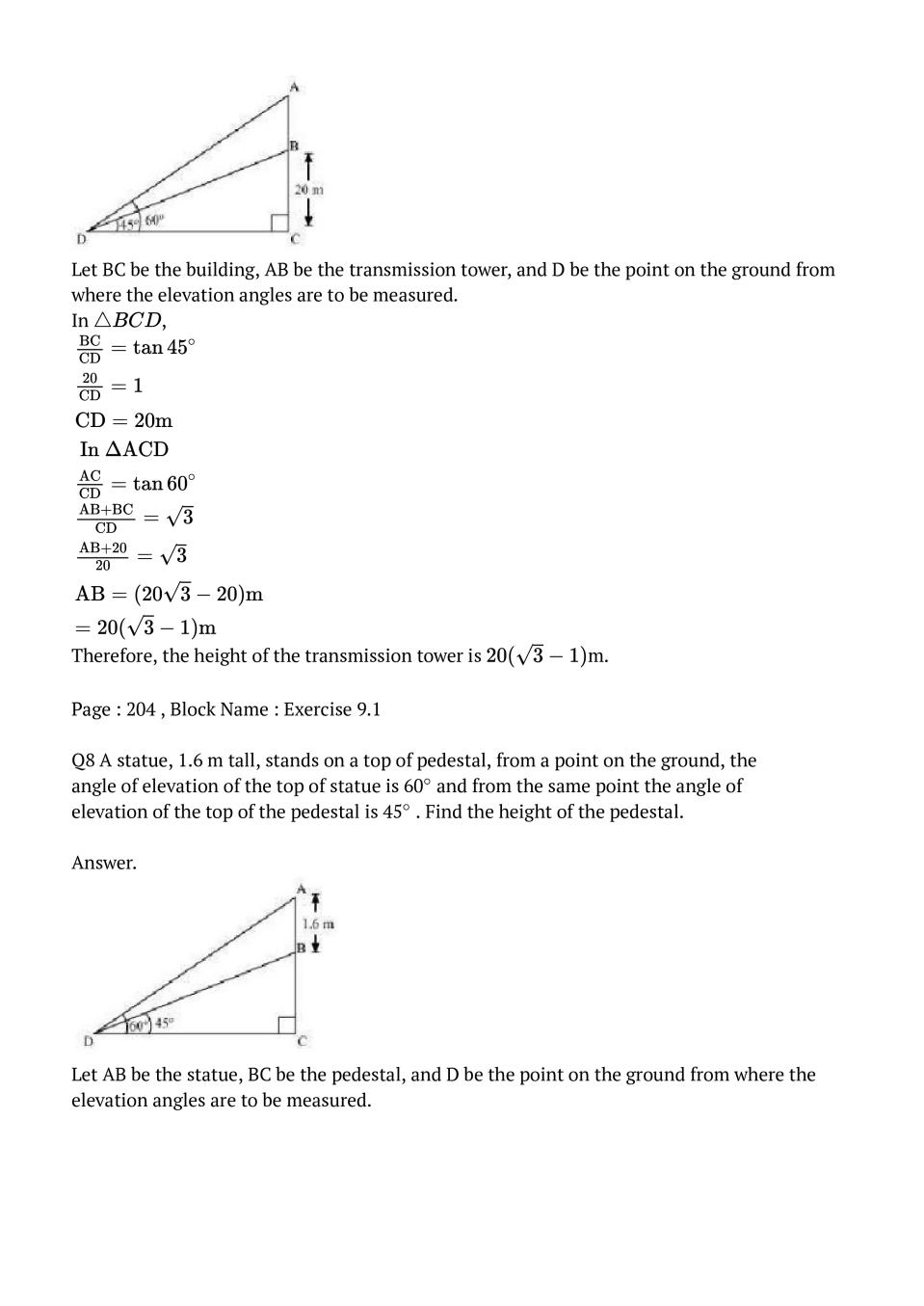
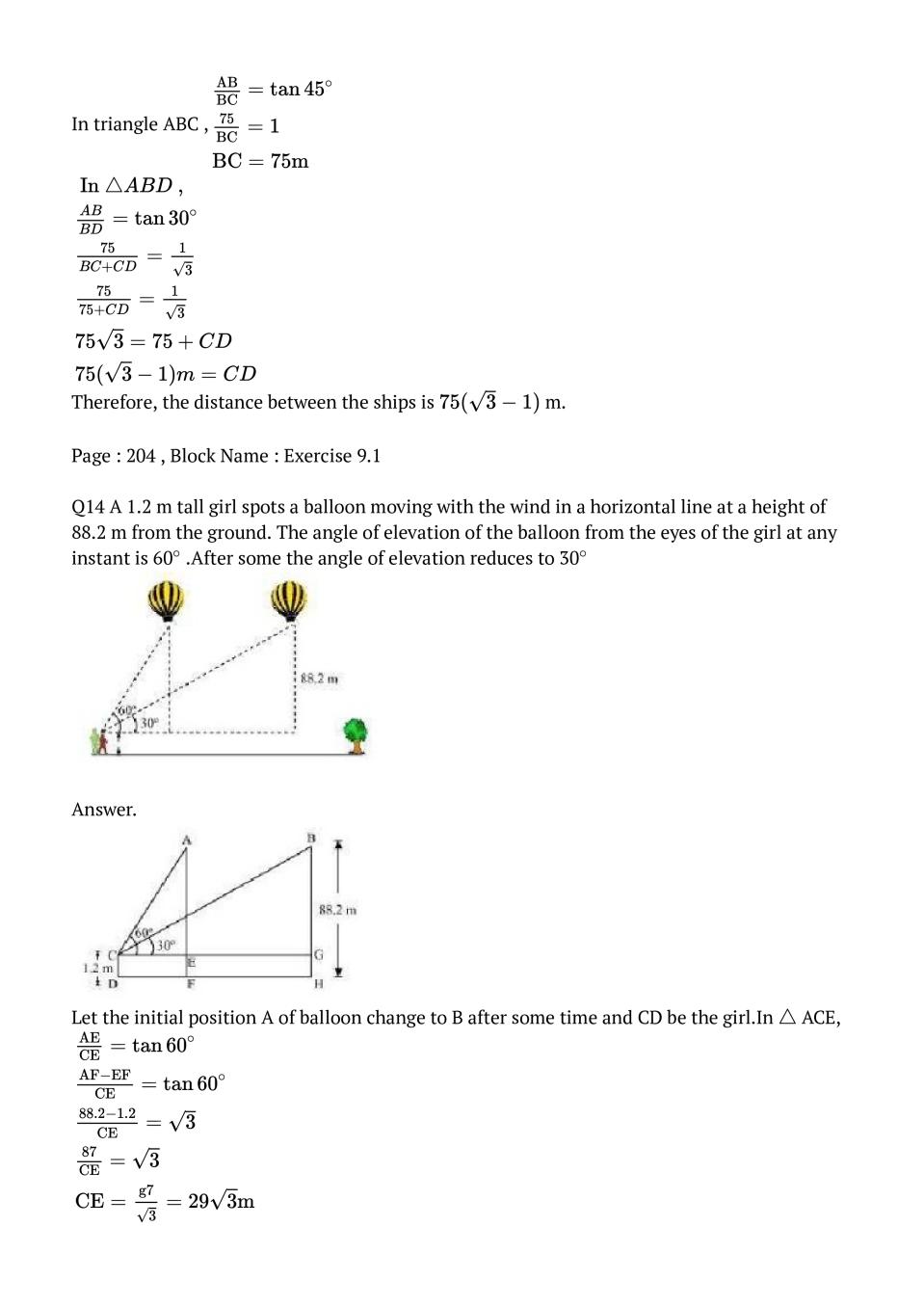
Q.3: A contractor plans to install two slides for the children to play in a park. For the children below the age of 5 years, she prefers to have a slide whose top is at a height of 1.5 m, and is inclined at an angle of 30\(^{\circ}\) to the ground, where as for the elder children she wants to have a steep side at a height of 3 m, and inclined at an angle of 60\(^{\circ}\) to the ground. What should be the length of the slide in each case?
Ans : It can be observed that AC and PR are the slides for younger and elder children respectively.\(\begin{array}{l}{\text { In } \triangle A B C,} \\ {\frac{A B}{A C}=\sin 30^{\circ}} \\ {\frac{1.5}{A C}=\frac{1}{2}} \\ {A C=3 \mathrm{m}}\end{array}\)
\(\begin{array}{l}{\text { In } \Delta \mathrm{PQR}_{,}} \\ {\frac{\mathrm{PQ}}{\mathrm{PR}}=\sin 60} \\ {\frac{3}{\mathrm{PR}}=\frac{\sqrt{3}}{2}} \\ {\mathrm{PR}=\frac{6}{\sqrt{3}}=2 \sqrt{3} \mathrm{m}}\end{array}\) Therefore, the length of these slides are 3 m and \(2 \sqrt{3} \mathrm{m}\).
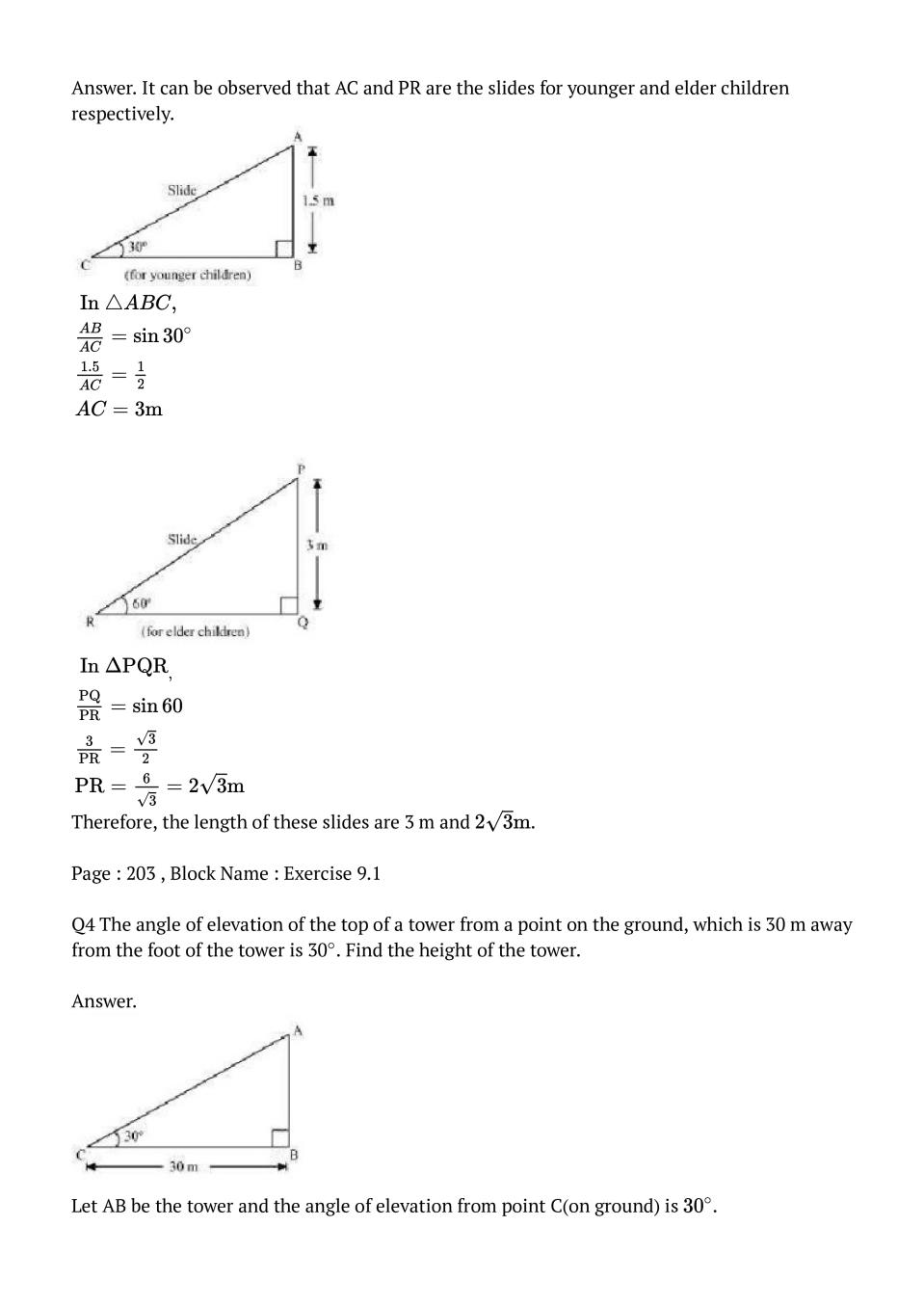
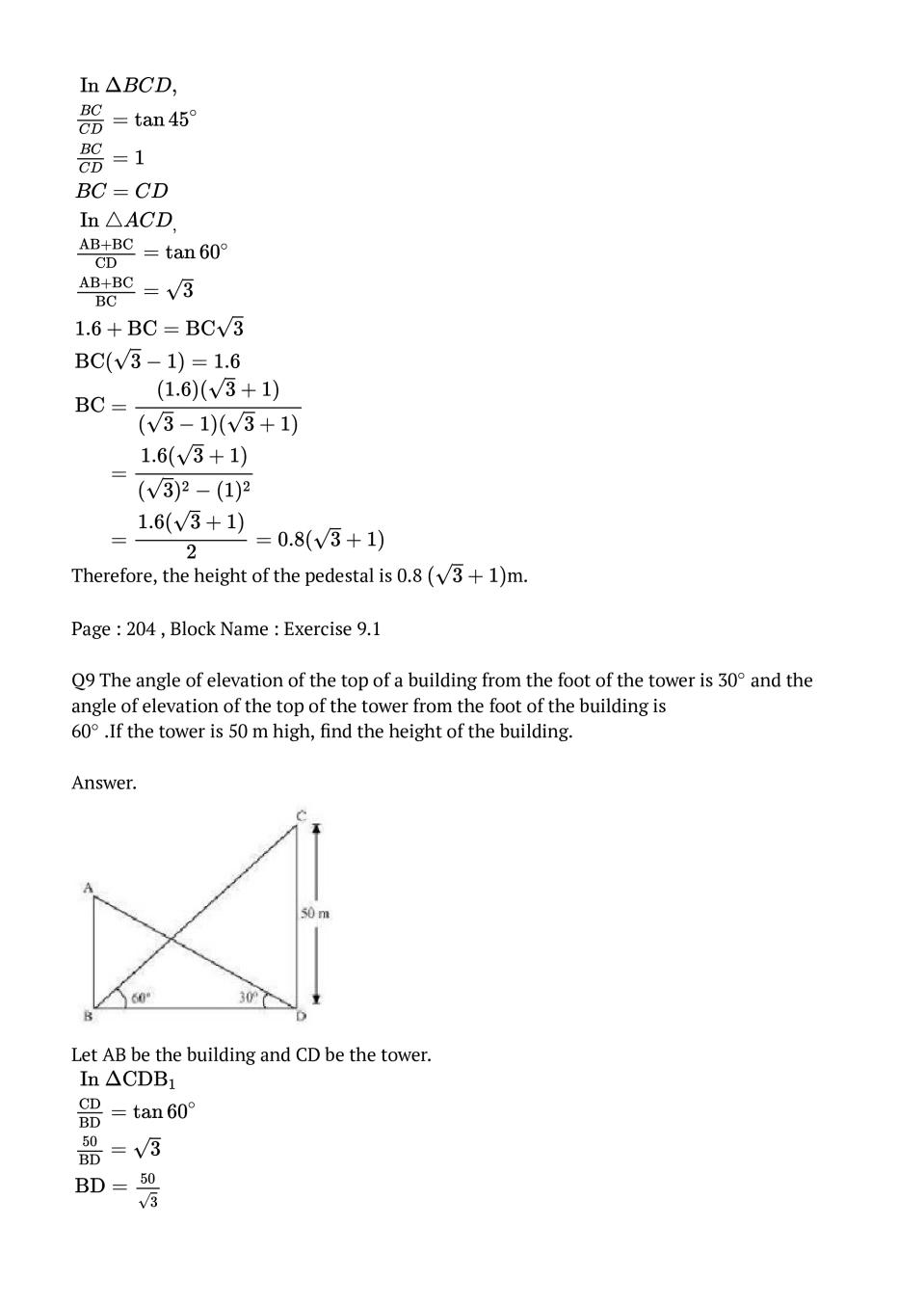
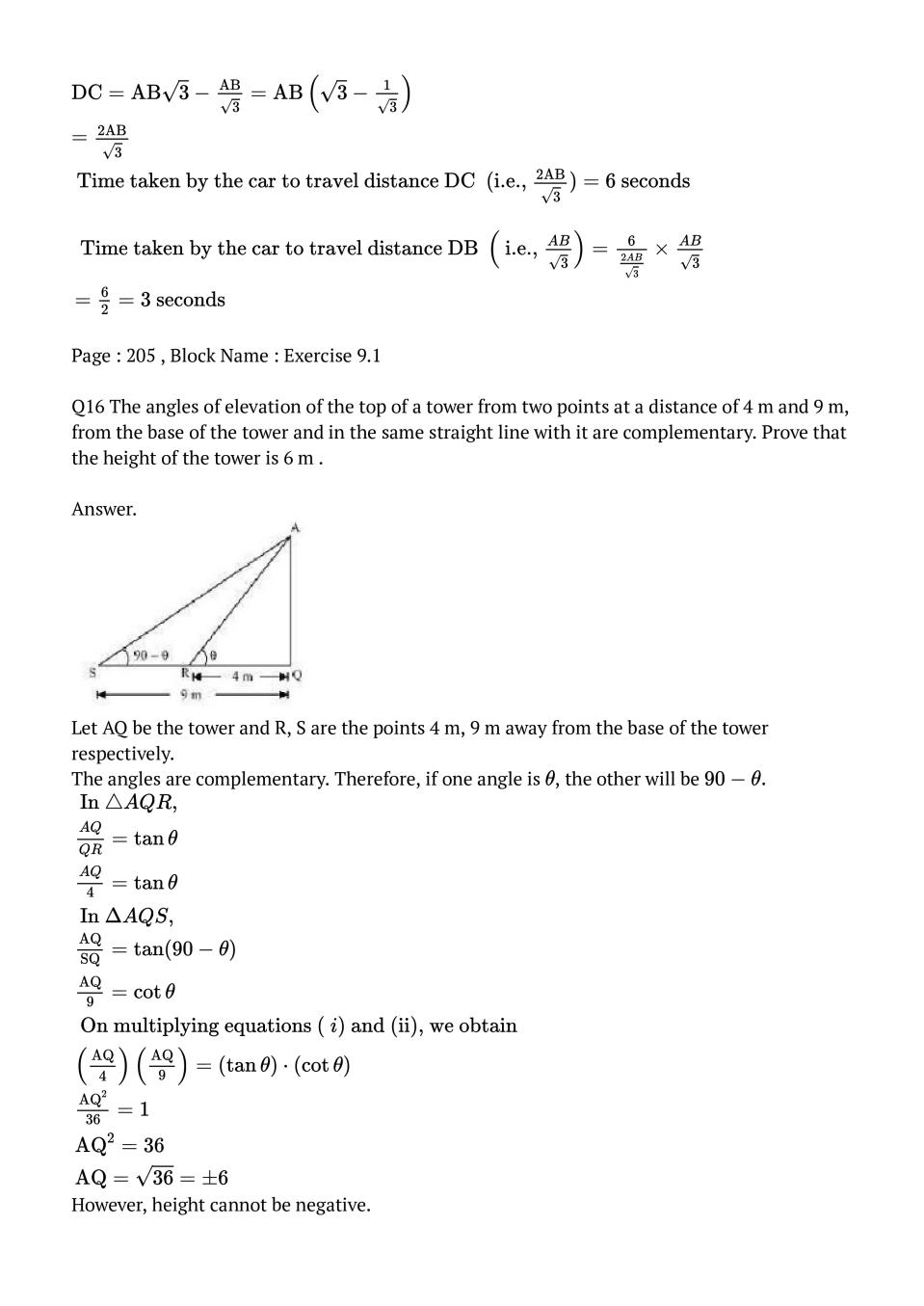
Q.4: The angle of elevation of the top of a tower from a point on the ground, which is 30 m away from the foot of the tower is 30\(^{\circ}\). Find the height of the tower.
Ans :Let AB be the tower and the angle of elevation from point C(on ground) is \(30^{\circ}\). In \(\triangle A B C\), \(\begin{array}{l}{\frac{\mathrm{AB}}{\mathrm{BC}}=\tan 30^{\circ}} \\ {\frac{\mathrm{AB}}{30}=\frac{1}{\sqrt{3}}} \\ {\mathrm{AB}=\frac{30}{\sqrt{3}}=10 \sqrt{3} \mathrm{m}}\end{array}\) Therefore, the height of the tower is \(10 \sqrt{3} \mathrm{m}\).
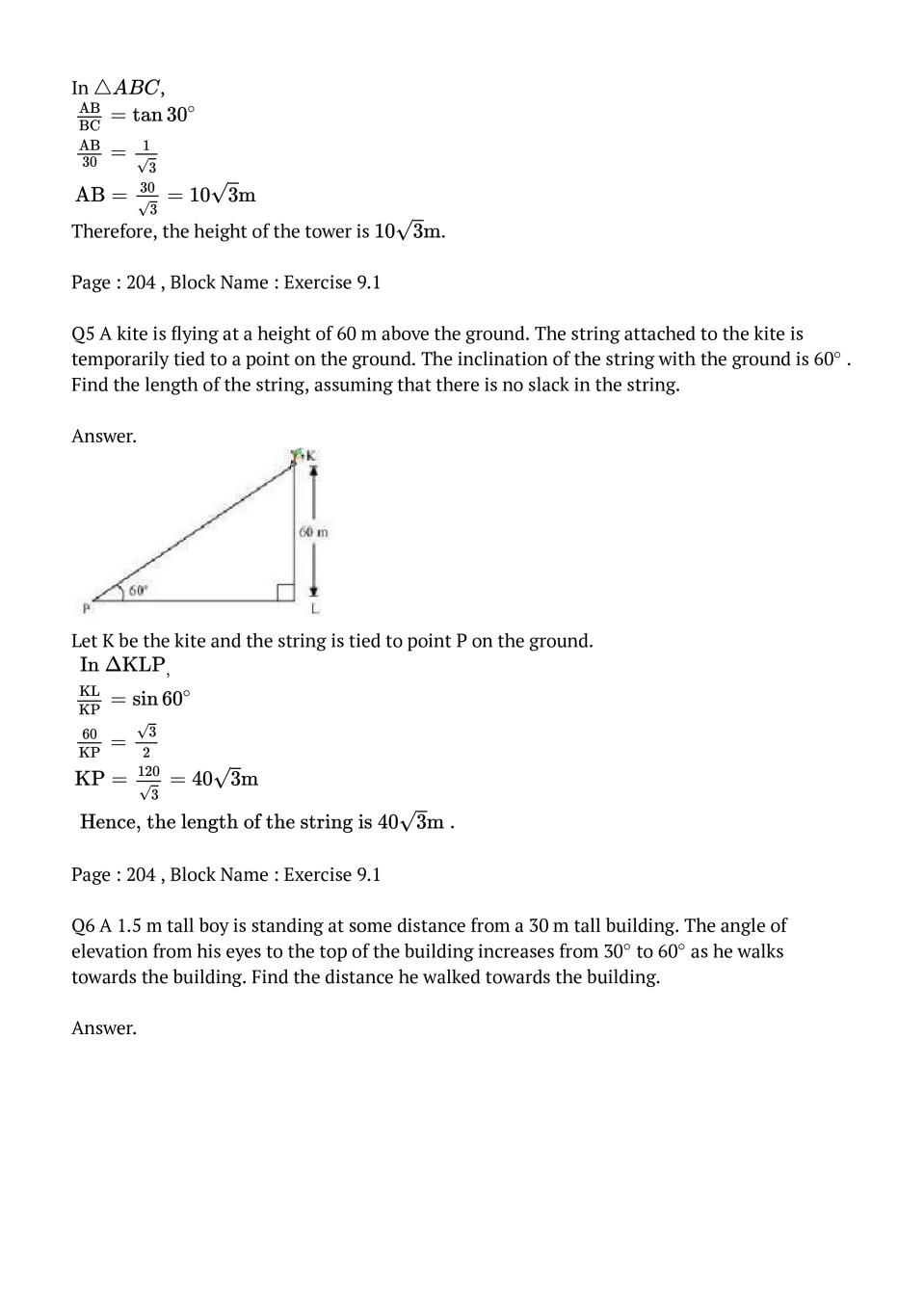
Q.5: A kite is flying at a height of 60 m above the ground. The string attached to the kite is temporarily tied to a point on the ground. The inclination of the string with the ground is 60\(^{\circ}\) . Find the length of the string, assuming that there is no slack in the string.
Ans :Let K be the kite and the string is tied to point P on the ground. \(\begin{array}{l}{\text { In } \Delta \mathrm{KLP}_{,}} \\ {\frac{\mathrm{KL}}{\mathrm{KP}}=\sin 60^{\circ}} \\ {\frac{60}{\mathrm{KP}}=\frac{\sqrt{3}}{2}} \\ {\mathrm{KP}=\frac{120}{\sqrt{3}}=40 \sqrt{3} \mathrm{m}} \\ {\text { Hence, the length of the string is } 40 \sqrt{3} \mathrm{m} \text { . }}\end{array}\)
NCERT / CBSE Book for Class 10 Maths
You can download the NCERT Book for Class 10 Maths in PDF format for free. Otherwise you can also buy it easily online.
- Click here for NCERT Book for Class 10 Maths
- Click here to buy NCERT Book for Class 10 Maths
All NCERT Solutions Class 10
- NCERT Solutions for Class 10 English
- NCERT Solutions for Class 10 Hindi
- NCERT Solutions for Class 10 Maths
- NCERT Solutions for Class 10 Science
- NCERT Solutions for Class 10 Social Science
- NCERT Solutions for Class 10 Sanskrit
All NCERT Solutions
You can also check out NCERT Solutions of other classes here. Click on the class number below to go to relevant NCERT Solutions of Class 1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12.
| Class 4 | Class 5 | Class 6 |
| Class 7 | Class 8 | Class 9 |
| Class 10 | Class 11 | Class 12 |
Download the NCERT Solutions app for quick access to NCERT Solutions Class 10 Maths Chapter 9 Some Applications of Trigonometry. It will help you stay updated with relevant study material to help you top your class!
The post NCERT Solutions for Class 10 Maths Chapter 9 Some Applications of Trigonometry appeared first on AglaSem Schools.
from AglaSem Schools https://ift.tt/3u8CgwI
https://ift.tt/2QxNvQM https://ift.tt/2QxNvQM





إرسال تعليق